Šis straipsnis yra apie pirmojo ir antrojo tipo kreivinius integralus .
Pirmojo tipo kreivinis integralas [ keisti ] Pirmojo tipo kreivinis integralas naudojamas dvimačio ar trimačio lanko masės apskaičiavimui. Galima apskaičiuoti masę, kai ji pastovi ar kai kinta pagal tam tikrą funkciją. Jeigu masė pastovi, tai jos skaičiavimas sutampa su lanko ilgio skaičiavimu.
d
s
=
1
+
y
′
2
d
x
,
{\displaystyle ds={\sqrt {1+y'^{2}}}dx,}
L apibrėžta lygtimi y=y(x), o
a
≤
x
≤
b
.
{\displaystyle a\leq x\leq b.}
∫
L
f
(
x
,
y
)
d
s
=
∫
a
b
f
(
x
,
y
(
x
)
)
1
+
(
y
′
(
x
)
)
2
d
x
.
{\displaystyle \int _{L}f(x,y)ds=\int _{a}^{b}f(x,y(x)){\sqrt {1+(y'(x))^{2}}}dx.}
Kai kreivė L apibrėžta parametrinėmis lygtimis
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
y
=
y
(
t
)
,
{\displaystyle y=y(t),}
t
∈
[
t
0
;
T
]
,
{\displaystyle t\in [t_{0};T],}
d
s
=
x
t
′
2
+
y
t
′
2
d
t
,
{\displaystyle ds={\sqrt {x_{t}'^{2}+y_{t}'^{2}}}dt,}
∫
L
f
(
x
,
y
)
d
s
=
∫
t
0
T
f
(
x
(
t
)
,
y
(
t
)
)
x
t
′
2
+
y
t
′
2
d
t
.
{\displaystyle \int _{L}f(x,y)ds=\int _{t_{0}}^{T}f(x(t),y(t)){\sqrt {x_{t}'^{2}+y_{t}'^{2}}}dt.}
Kai prametrinėmis lygtimis
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
y
=
y
(
t
)
,
{\displaystyle y=y(t),}
z
=
z
(
t
)
,
{\displaystyle z=z(t),}
t
∈
[
t
0
;
T
]
{\displaystyle t\in [t_{0};T]}
L , tai
∫
L
f
(
x
,
y
,
z
)
d
s
=
∫
t
0
T
f
(
x
(
t
)
,
y
(
t
)
,
z
(
t
)
)
x
t
′
2
+
y
t
′
2
+
z
t
′
2
d
t
.
{\displaystyle \int _{L}f(x,y,z)ds=\int _{t_{0}}^{T}f(x(t),y(t),z(t)){\sqrt {x_{t}'^{2}+y_{t}'^{2}+z_{t}'^{2}}}dt.}
Kai kreivė L polinėje koordinačių sistemoje apibrėžta lygtimi
ρ
=
ρ
(
ϕ
)
,
{\displaystyle \rho =\rho (\phi ),}
ϕ
∈
[
α
;
β
]
{\displaystyle \phi \in [\alpha ;\beta ]}
d
s
=
ρ
2
+
ρ
′
2
d
ϕ
{\displaystyle ds={\sqrt {\rho ^{2}+\rho '^{2}}}d\phi }
∫
L
f
(
x
,
y
)
d
s
=
∫
α
β
f
(
ρ
cos
ϕ
,
ρ
sin
ϕ
)
ρ
2
+
ρ
′
2
d
ϕ
.
{\displaystyle \int _{L}f(x,y)ds=\int _{\alpha }^{\beta }f(\rho \cos \phi ,\rho \sin \phi ){\sqrt {\rho ^{2}+\rho '^{2}}}d\phi .}
Apskaičiuokime integralą
∫
L
(
x
+
y
)
d
s
,
{\displaystyle \int _{L}(x+{\sqrt {y}})ds,}
L - prabolės
y
=
1
2
x
2
{\displaystyle y={1 \over 2}x^{2}}
Remdamiesi sąlyga
y
=
1
2
x
2
,
{\displaystyle y={1 \over 2}x^{2},}
d
s
=
1
+
x
2
.
{\displaystyle ds={\sqrt {1+x^{2}}}.}
∫
L
(
x
+
y
)
d
s
=
∫
0
1
(
x
+
1
2
x
)
1
+
x
2
d
x
=
(
1
+
1
2
)
∫
0
1
x
1
+
x
2
d
x
=
{\displaystyle \int _{L}(x+{\sqrt {y}})ds=\int _{0}^{1}(x+{1 \over {\sqrt {2}}}x){\sqrt {1+x^{2}}}dx=(1+{1 \over {\sqrt {2}}})\int _{0}^{1}x{\sqrt {1+x^{2}}}dx=}
=
2
+
1
2
∫
0
1
1
+
x
2
d
(
1
+
x
2
)
2
=
2
+
1
3
2
(
1
+
x
2
)
3
|
0
1
=
2
+
1
3
2
(
2
2
−
1
)
=
4
−
1
+
2
2
−
2
3
2
=
3
+
2
3
2
=
1
6
(
2
+
3
2
)
,
{\displaystyle ={{\sqrt {2}}+1 \over {\sqrt {2}}}\int _{0}^{1}{\sqrt {1+x^{2}}}{d(1+x^{2}) \over 2}={{\sqrt {2}}+1 \over 3{\sqrt {2}}}{\sqrt {(1+x^{2})^{3}}}|_{0}^{1}={{\sqrt {2}}+1 \over 3{\sqrt {2}}}(2{\sqrt {2}}-1)={4-1+2{\sqrt {2}}-{\sqrt {2}} \over 3{\sqrt {2}}}={3+{\sqrt {2}} \over 3{\sqrt {2}}}={1 \over 6}(2+3{\sqrt {2}}),}
kur
d
(
1
+
x
2
)
=
2
x
d
x
;
{\displaystyle d(1+x^{2})=2xdx;}
d
x
=
d
(
1
+
x
2
)
2
x
.
{\displaystyle dx={d(1+x^{2}) \over 2x}.}
Apskaičiuosime kreivinį integralą
∫
A
B
y
d
l
,
{\displaystyle \int _{AB}y\;dl,}
AB - parabolės
y
2
=
2
x
{\displaystyle y^{2}=2x}
Turime
y
=
2
x
,
y
′
=
1
2
x
,
d
l
=
1
+
y
′
2
d
x
=
1
+
1
2
x
d
x
.
{\displaystyle y={\sqrt {2x}},\;y'={1 \over {\sqrt {2x}}},\;dl={\sqrt {1+y'^{2}}}dx={\sqrt {1+{1 \over 2x}}}dx.}
∫
A
B
y
d
l
=
∫
0
2
2
x
1
+
1
2
x
d
x
=
∫
0
2
2
x
+
1
d
x
=
∫
0
2
2
x
+
1
d
(
2
x
+
1
)
2
=
(
2
x
+
1
)
3
2
3
|
0
2
=
5
5
−
1
3
.
{\displaystyle \int _{AB}ydl=\int _{0}^{2}{\sqrt {2x}}{\sqrt {1+{1 \over 2x}}}dx=\int _{0}^{2}{\sqrt {2x+1}}dx=\int _{0}^{2}{\sqrt {2x+1}}{d(2x+1) \over 2}={(2x+1)^{3 \over 2} \over 3}|_{0}^{2}={5{\sqrt {5}}-1 \over 3}.}
Apskaičiuokime kreivės
y
2
=
4
9
x
3
,
{\displaystyle y^{2}={4 \over 9}x^{3},}
(
x
∈
[
3
;
8
]
)
{\displaystyle (x\in [3;8])}
L masę, kai tankis kreivės taške yra tiesiog proporcingas to taško ordinatei (y ) ir atvirkščiai proporcingas kvadratinei šakniai iš to taško abscisės (
1
/
x
1
/
2
{\displaystyle 1/x^{1/2}}
(
4
;
16
3
)
{\displaystyle (4;{16 \over 3})}
Kreivės lanko masę, kai to lanko tankis lygus
γ
(
x
,
y
)
{\displaystyle \gamma (x,y)}
m
=
∫
L
γ
(
x
,
y
)
d
s
.
{\displaystyle m=\int _{L}\gamma (x,y)ds.}
Pagal uždavinio sąlyga, tankis lygus
γ
(
x
,
y
)
=
k
y
x
;
{\displaystyle \gamma (x,y)={ky \over {\sqrt {x}}};}
k - proporcingumo koeficientas. Kadangi
γ
=
8
{\displaystyle \gamma =8}
x
=
4
,
{\displaystyle x=4,}
y
=
16
3
,
{\displaystyle y={16 \over 3},}
8
=
k
16
3
4
=
8
k
3
{\displaystyle 8=k{{16 \over 3} \over {\sqrt {4}}}={8k \over 3}}
m
=
∫
L
γ
(
x
,
y
)
d
s
=
3
∫
L
y
x
d
s
.
{\displaystyle m=\int _{L}\gamma (x,y)ds=3\int _{L}{y \over {\sqrt {x}}}ds.}
Norėdami apskaičiuoti šį integralą, taikysime pirmą formulę. Iš sąlygos
y
=
2
3
x
x
{\displaystyle y={2 \over 3}x{\sqrt {x}}}
y
′
=
x
{\displaystyle y'={\sqrt {x}}}
d
s
=
1
+
(
y
′
)
2
d
x
=
1
+
x
d
x
.
{\displaystyle ds={\sqrt {1+(y')^{2}}}dx={\sqrt {1+x}}dx.}
Tuomet
m
=
3
∫
3
8
2
3
x
x
x
1
+
x
d
x
=
2
∫
3
8
x
1
+
x
d
x
=
4
∫
2
3
(
t
2
−
1
)
t
2
d
t
=
4
(
t
5
5
−
t
3
3
)
|
2
3
=
{\displaystyle m=3\int _{3}^{8}{{2 \over 3}x{\sqrt {x}} \over {\sqrt {x}}}{\sqrt {1+x}}dx=2\int _{3}^{8}x{\sqrt {1+x}}dx=4\int _{2}^{3}(t^{2}-1)t^{2}dt=4({t^{5} \over 5}-{t^{3} \over 3})|_{2}^{3}=}
=
4
[
243
5
−
9
−
(
32
5
−
8
3
)
]
=
4
(
211
5
−
19
3
)
=
2152
15
(
g
)
=
143.4
(
6
)
(
g
)
,
{\displaystyle =4[{243 \over 5}-9-({32 \over 5}-{8 \over 3})]=4({211 \over 5}-{19 \over 3})={2152 \over 15}\;(g)=143.4(6)\;(g),}
kur
1
+
x
=
t
,
{\displaystyle {\sqrt {1+x}}=t,}
1
+
x
=
t
2
,
{\displaystyle 1+x=t^{2},}
x
=
t
2
−
1
,
{\displaystyle x=t^{2}-1,}
d
x
=
2
t
d
t
,
{\displaystyle dx=2tdt,}
t
1
=
2
,
{\displaystyle t_{1}=2,}
t
2
=
3.
{\displaystyle t_{2}=3.}
Arba galėjome apskaičiuoti integruodami dalimis :
m
=
2
∫
3
8
x
1
+
x
d
x
=
2
x
⋅
2
3
(
1
+
x
)
3
2
|
3
8
−
2
∫
3
8
2
3
(
1
+
x
)
3
2
d
x
=
{\displaystyle m=2\int _{3}^{8}x{\sqrt {1+x}}dx=2x\cdot {2 \over 3}(1+x)^{3 \over 2}|_{3}^{8}-2\int _{3}^{8}{2 \over 3}(1+x)^{3 \over 2}dx=}
=
4
3
x
(
1
+
x
)
1
+
x
|
3
8
−
4
3
⋅
2
5
(
1
+
x
)
5
2
|
3
8
=
(
32
3
⋅
9
⋅
3
−
4
⋅
4
⋅
2
)
−
(
8
15
⋅
3
5
−
8
15
⋅
2
5
)
=
{\displaystyle ={4 \over 3}x(1+x){\sqrt {1+x}}|_{3}^{8}-{4 \over 3}\cdot {2 \over 5}(1+x)^{5 \over 2}|_{3}^{8}=({32 \over 3}\cdot 9\cdot 3-4\cdot 4\cdot 2)-({8 \over 15}\cdot 3^{5}-{8 \over 15}\cdot 2^{5})=}
=
(
288
−
32
)
−
(
1994
15
−
256
15
)
=
256
−
1688
15
=
2152
15
(
g
)
=
143.4
(
6
)
(
g
)
,
{\displaystyle =(288-32)-({1994 \over 15}-{256 \over 15})=256-{1688 \over 15}={2152 \over 15}\;(g)=143.4(6)\;(g),}
kur
u
=
x
,
{\displaystyle u=x,}
d
v
=
1
+
x
,
{\displaystyle dv={\sqrt {1+x}},}
d
u
=
d
x
,
{\displaystyle du=dx,}
v
=
∫
(
1
+
x
)
0.5
d
x
=
2
3
(
1
+
x
)
3
2
.
{\displaystyle v=\int (1+x)^{0.5}dx={2 \over 3}(1+x)^{3 \over 2}.}
cikloidė Apskaičiuokime integralą
∫
L
x
d
s
,
{\displaystyle \int _{L}xds,}
L - pirmoji cikloidės
x
=
a
(
t
−
sin
t
)
,
{\displaystyle x=a(t-\sin t),}
y
=
a
(
1
−
cos
t
)
{\displaystyle y=a(1-\cos t)}
Taikome antrą formulę. Randame:
x
t
′
=
a
(
1
−
cos
t
)
,
{\displaystyle x_{t}'=a(1-\cos t),}
y
t
′
=
a
sin
t
,
{\displaystyle y_{t}'=a\sin t,}
d
s
=
x
t
′
2
+
y
t
′
2
d
t
=
a
2
(
1
−
cos
t
)
2
+
a
2
sin
2
t
d
t
=
a
1
−
2
cos
t
+
cos
2
+
sin
2
t
d
t
=
{\displaystyle ds={\sqrt {x_{t}'^{2}+y_{t}'^{2}}}dt={\sqrt {a^{2}(1-\cos t)^{2}+a^{2}\sin ^{2}t}}dt=a{\sqrt {1-2\cos t+\cos ^{2}+\sin ^{2}t}}dt=}
=
a
2
−
2
cos
t
d
t
=
a
2
−
2
(
1
−
2
sin
2
t
2
)
d
t
=
2
a
sin
t
2
d
t
.
{\displaystyle =a{\sqrt {2-2\cos t}}dt=a{\sqrt {2-2(1-2\sin ^{2}{t \over 2})}}dt=2a\sin {t \over 2}dt.}
∫
L
d
s
=
2
a
2
∫
0
2
π
(
t
−
sin
t
)
sin
t
2
d
t
=
2
a
2
(
∫
0
2
π
t
sin
t
2
d
t
−
∫
0
2
π
sin
t
sin
t
2
d
t
)
.
{\displaystyle \int _{L}ds=2a^{2}\int _{0}^{2\pi }(t-\sin t)\sin {t \over 2}dt=2a^{2}(\int _{0}^{2\pi }t\sin {t \over 2}dt-\int _{0}^{2\pi }\sin t\sin {t \over 2}dt).}
Pirmąjį integralą integruojame dalimis, pažymėdami
u
=
t
,
{\displaystyle u=t,}
d
v
=
sin
t
2
d
t
,
{\displaystyle dv=\sin {t \over 2}dt,}
d
u
=
d
t
,
{\displaystyle du=dt,}
v
=
∫
sin
t
2
d
t
=
−
2
cos
t
2
,
{\displaystyle v=\int \sin {t \over 2}dt=-2\cos {t \over 2},}
∫
0
2
π
t
sin
t
2
d
t
=
−
2
(
t
cos
t
2
)
|
0
2
π
+
2
∫
0
2
π
cos
t
2
d
t
=
−
2
(
2
π
(
−
1
)
)
+
4
sin
t
2
|
0
2
π
=
4
π
.
{\displaystyle \int _{0}^{2\pi }t\sin {t \over 2}dt=-2(t\cos {t \over 2})|_{0}^{2\pi }+2\int _{0}^{2\pi }\cos {t \over 2}dt=-2(2\pi (-1))+4\sin {t \over 2}|_{0}^{2\pi }=4\pi .}
Antrąjį integralą apskaičiuojame taikydami formulę
sin
t
sin
t
2
=
(
2
sin
t
2
cos
t
2
)
sin
t
2
=
2
sin
2
t
2
cos
t
2
,
{\displaystyle \sin t\sin {t \over 2}=(2\sin {t \over 2}\cos {t \over 2})\sin {t \over 2}=2\sin ^{2}{t \over 2}\cos {t \over 2},}
d
(
sin
t
2
)
=
cos
t
2
d
(
t
2
)
,
d
(
t
2
)
=
1
2
d
t
,
d
t
=
2
d
(
t
2
)
,
{\displaystyle d(\sin {t \over 2})=\cos {t \over 2}d({t \over 2}),\;d({t \over 2})={1 \over 2}dt,\;dt=2d({t \over 2}),}
∫
0
2
π
sin
t
sin
t
2
d
t
=
∫
0
2
π
2
sin
2
t
2
cos
t
2
2
d
(
t
2
)
=
4
∫
0
2
π
sin
2
t
2
d
(
sin
t
2
)
=
4
3
sin
3
t
2
|
0
2
π
=
0.
{\displaystyle \int _{0}^{2\pi }\sin t\sin {t \over 2}dt=\int _{0}^{2\pi }2\sin ^{2}{t \over 2}\cos {t \over 2}\;2d({t \over 2})=4\int _{0}^{2\pi }\sin ^{2}{t \over 2}\;d(\sin {t \over 2})={4 \over 3}\sin ^{3}{t \over 2}|_{0}^{2\pi }=0.}
Todėl bendras integralas lygus:
∫
L
x
d
s
=
2
a
2
(
4
π
−
0
)
=
8
π
a
2
.
{\displaystyle \int _{L}xds=2a^{2}(4\pi -0)=8\pi a^{2}.}
Reikia apskaičiuoti integralą
∫
A
B
(
x
2
+
y
2
+
z
2
)
d
s
{\displaystyle \int _{AB}(x^{2}+y^{2}+z^{2})ds}
x
=
cos
t
,
{\displaystyle x=\cos t,}
y
=
sin
t
,
{\displaystyle y=\sin t,}
z
=
t
,
{\displaystyle z=t,}
0
≤
t
≤
2
π
.
{\displaystyle 0\leq t\leq 2\pi .}
Pagal trečią formulę gauname:
∫
A
B
(
x
2
+
y
2
+
z
2
)
d
s
=
∫
0
2
π
(
cos
2
t
+
sin
2
t
+
t
2
)
(
−
sin
t
)
2
+
(
cos
t
)
2
+
1
d
t
=
2
∫
0
2
π
(
1
+
t
2
)
d
t
=
{\displaystyle \int _{AB}(x^{2}+y^{2}+z^{2})ds=\int _{0}^{2\pi }(\cos ^{2}t+\sin ^{2}t+t^{2}){\sqrt {(-\sin t)^{2}+(\cos t)^{2}+1}}dt={\sqrt {2}}\int _{0}^{2\pi }(1+t^{2})dt=}
=
2
(
t
+
t
3
3
|
0
2
π
=
2
2
π
(
1
+
4
π
2
3
)
.
{\displaystyle ={\sqrt {2}}(t+{t^{3} \over 3}|_{0}^{2\pi }=2{\sqrt {2}}\pi (1+{4\pi ^{2} \over 3}).}
Apskaičiuosime integralą
∫
A
B
x
2
d
s
,
{\displaystyle \int _{AB}x^{2}ds,}
AB - dalis logoritminės kreivės
y
=
ln
x
{\displaystyle y=\ln x}
x
=
1
{\displaystyle x=1}
x
=
2.
{\displaystyle x=2.}
Pagal pirmą formulę
∫
A
B
x
2
d
s
=
∫
1
2
x
2
1
+
1
x
2
d
x
=
∫
1
2
x
x
2
+
1
d
x
=
∫
1
2
x
x
2
+
1
d
(
x
2
+
1
)
2
x
=
1
3
(
1
+
x
2
)
3
2
|
x
=
1
x
=
2
=
{\displaystyle \int _{AB}x^{2}ds=\int _{1}^{2}x^{2}{\sqrt {1+{1 \over x^{2}}}}dx=\int _{1}^{2}x{\sqrt {x^{2}+1}}dx=\int _{1}^{2}x{\sqrt {x^{2}+1}}{d(x^{2}+1) \over 2x}={1 \over 3}(1+x^{2})^{3 \over 2}|_{x=1}^{x=2}=}
=
1
3
(
5
5
−
2
2
)
,
{\displaystyle ={1 \over 3}(5{\sqrt {5}}-2{\sqrt {2}}),}
d
(
x
2
+
1
)
=
2
x
d
x
.
{\displaystyle d(x^{2}+1)=2xdx.}
Apskaičiuosime kreivinį integralą
∫
A
B
y
2
d
l
,
{\displaystyle \int _{AB}y^{2}dl,}
AB - dalis apskritimo
x
=
a
cos
t
,
{\displaystyle x=a\cos t,}
y
=
a
sin
t
,
{\displaystyle y=a\sin t,}
0
≤
t
≤
π
2
.
{\displaystyle 0\leq t\leq {\pi \over 2}.}
Kadangi
y
2
=
a
2
sin
2
t
,
{\displaystyle y^{2}=a^{2}\sin ^{2}t,}
d
l
=
a
2
sin
2
t
+
a
2
cos
2
t
d
t
=
a
d
t
,
{\displaystyle dl={\sqrt {a^{2}\sin ^{2}t+a^{2}\cos ^{2}t}}dt=a\;dt,}
∫
A
B
y
2
d
l
=
∫
0
π
/
2
a
2
sin
2
t
⋅
a
d
t
=
a
3
2
∫
0
π
2
(
1
−
cos
(
2
t
)
)
d
t
=
a
3
2
(
t
−
sin
(
2
t
)
2
)
|
0
π
2
=
a
3
π
4
.
{\displaystyle \int _{AB}y^{2}dl=\int _{0}^{\pi /2}a^{2}\sin ^{2}t\cdot a\;dt={a^{3} \over 2}\int _{0}^{\pi \over 2}(1-\cos(2t))dt={a^{3} \over 2}(t-{\sin(2t) \over 2})|_{0}^{\pi \over 2}={a^{3}\pi \over 4}.}
Apskaičiuokime
∫
L
(
x
+
y
)
d
s
,
{\displaystyle \int _{L}(x+y)ds,}
L - apskritimas
x
2
+
y
2
=
a
y
,
{\displaystyle x^{2}+y^{2}=ay,}
(
a
>
0
)
.
{\displaystyle (a>0).}
Integralą apskaičiuokime, Dekatro koordinates pakeitę polinėmis. Kreivės L lygtis šioje koordinačių sistemoje yra
ρ
=
a
sin
ϕ
,
{\displaystyle \rho =a\sin \phi ,}
ϕ
∈
[
0
;
π
]
.
{\displaystyle \phi \in [0;\pi ].}
ρ
′
=
a
cos
ϕ
,
{\displaystyle \rho '=a\cos \phi ,}
d
s
=
ρ
2
+
ρ
ϕ
′
2
d
ϕ
=
a
sin
2
ϕ
+
a
cos
2
ϕ
d
ϕ
=
a
d
ϕ
.
{\displaystyle ds={\sqrt {\rho ^{2}+\rho _{\phi }'^{2}}}d\phi ={\sqrt {a\sin ^{2}\phi +a\cos ^{2}\phi }}d\phi =a\;d\phi .}
Tuomet
∫
L
(
x
+
y
)
d
s
=
a
∫
0
π
(
ρ
sin
ϕ
+
ρ
cos
ϕ
)
d
ϕ
=
a
2
∫
0
π
(
sin
2
ϕ
+
sin
ϕ
cos
ϕ
)
d
ϕ
=
{\displaystyle \int _{L}(x+y)ds=a\int _{0}^{\pi }(\rho \sin \phi +\rho \cos \phi )d\phi =a^{2}\int _{0}^{\pi }(\sin ^{2}\phi +\sin \phi \cos \phi )d\phi =}
=
a
2
∫
0
π
(
1
−
cos
(
2
ϕ
)
2
+
sin
(
2
ϕ
)
2
)
d
ϕ
=
a
2
π
2
−
a
2
4
sin
(
2
ϕ
)
|
0
π
−
a
2
4
cos
(
2
ϕ
)
|
0
π
=
a
2
π
2
−
0
−
0
=
π
a
2
2
.
{\displaystyle =a^{2}\int _{0}^{\pi }({1-\cos(2\phi ) \over 2}+{\sin(2\phi ) \over 2})d\phi ={a^{2}\pi \over 2}-{a^{2} \over 4}\sin(2\phi )|_{0}^{\pi }-{a^{2} \over 4}\cos(2\phi )|_{0}^{\pi }={a^{2}\pi \over 2}-0-0={\pi a^{2} \over 2}.}
Kreivės lanko ilgis randamas pagal šitas formules:
d
s
=
1
+
y
′
2
d
x
,
{\displaystyle ds={\sqrt {1+y'^{2}}}dx,}
L apibrėžta lygtimi y=y(x), o
a
≤
x
≤
b
.
{\displaystyle a\leq x\leq b.}
∫
L
d
s
=
∫
a
b
1
+
(
f
′
(
x
)
)
2
d
x
=
∫
a
b
1
+
(
y
′
)
2
d
x
.
(
2
)
{\displaystyle \int _{L}ds=\int _{a}^{b}{\sqrt {1+(f'(x))^{2}}}dx=\int _{a}^{b}{\sqrt {1+(y')^{2}}}dx.\quad (2)}
Kai kreivė L apibrėžta parametrinėmis lygtimis
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
y
=
y
(
t
)
,
{\displaystyle y=y(t),}
t
∈
[
t
0
;
T
]
,
{\displaystyle t\in [t_{0};T],}
d
s
=
x
t
′
2
+
y
t
′
2
d
t
,
{\displaystyle ds={\sqrt {x_{t}'^{2}+y_{t}'^{2}}}dt,}
∫
L
d
s
=
∫
t
0
T
(
x
t
′
)
2
+
(
y
t
′
)
2
d
t
.
{\displaystyle \int _{L}ds=\int _{t_{0}}^{T}{\sqrt {(x_{t}')^{2}+(y_{t}')^{2}}}dt.}
Elementariausias pavyzdis, kai reikia perrašyti funkcija
y
=
x
2
{\displaystyle y=x^{2}}
x
=
t
;
y
=
t
2
{\displaystyle x=t;\;y=t^{2}}
x
t
′
=
t
′
=
1
;
y
t
′
=
(
t
2
)
′
=
2
t
{\displaystyle x_{t}'=t'=1;\;y_{t}'=(t^{2})'=2t}
∫
t
0
T
(
x
t
′
)
2
+
(
y
t
′
)
2
d
t
=
∫
t
0
T
1
2
+
(
2
t
)
2
d
t
=
∫
t
0
T
1
+
4
⋅
t
2
d
t
.
{\displaystyle \int _{t_{0}}^{T}{\sqrt {(x_{t}')^{2}+(y_{t}')^{2}}}dt=\int _{t_{0}}^{T}{\sqrt {1^{2}+(2t)^{2}}}dt=\int _{t_{0}}^{T}{\sqrt {1+4\cdot t^{2}}}dt.}
Kai prametrinėmis lygtimis
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
y
=
y
(
t
)
,
{\displaystyle y=y(t),}
z
=
z
(
t
)
,
{\displaystyle z=z(t),}
t
∈
[
t
0
;
T
]
{\displaystyle t\in [t_{0};T]}
L , tai
∫
L
d
s
=
∫
t
0
T
(
x
t
′
)
2
+
(
y
t
′
)
2
+
(
z
t
′
)
2
d
t
.
{\displaystyle \int _{L}ds=\int _{t_{0}}^{T}{\sqrt {(x_{t}')^{2}+(y_{t}')^{2}+(z_{t}')^{2}}}dt.}
Kai kreivė L polinėje koordinačių sistemoje apibrėžta lygtimi
ρ
=
ρ
(
ϕ
)
,
{\displaystyle \rho =\rho (\phi ),}
ϕ
∈
[
α
;
β
]
{\displaystyle \phi \in [\alpha ;\beta ]}
d
s
=
ρ
2
+
ρ
′
2
d
ϕ
{\displaystyle ds={\sqrt {\rho ^{2}+\rho '^{2}}}d\phi }
∫
L
d
s
=
∫
α
β
ρ
2
+
(
ρ
′
)
2
d
ϕ
.
{\displaystyle \int _{L}ds=\int _{\alpha }^{\beta }{\sqrt {\rho ^{2}+(\rho ')^{2}}}d\phi .}
Kreivės lanko ilgio formulių išvedimas Rasime dabar kreivės lanko ilgį tuo atveju, kai kreivės užrašyta paramtetrinėmis lygtimis:
x
=
ϕ
(
t
)
,
y
=
ψ
(
t
)
(
α
≤
t
≤
β
)
(
4
)
,
{\displaystyle x=\phi (t),\;\;y=\psi (t)\;\;(\alpha \leq t\leq \beta )\quad (4),}
čia
ϕ
(
t
)
{\displaystyle \phi (t)}
ψ
(
t
)
{\displaystyle \psi (t)}
ϕ
′
(
t
)
{\displaystyle \phi '(t)}
y
=
f
(
x
)
,
{\displaystyle y=f(x),}
d
y
d
x
=
ψ
′
(
t
)
ϕ
′
(
t
)
.
{\displaystyle {\frac {dy}{dx}}={\frac {\psi '(t)}{\phi '(t)}}.}
Tegu
a
=
ϕ
(
α
)
,
b
=
ϕ
(
β
)
.
{\displaystyle a=\phi (\alpha ),\;b=\phi (\beta ).}
x
=
ϕ
(
t
)
,
d
x
=
ϕ
′
(
t
)
d
t
,
{\displaystyle x=\phi (t),\;\;dx=\phi '(t)\;dt,}
gausime:
s
=
∫
α
β
1
+
[
ψ
′
(
t
)
ϕ
′
(
t
)
]
2
ϕ
′
(
t
)
d
t
=
∫
α
β
[
ϕ
′
(
t
)
]
2
+
[
ψ
′
(
t
)
]
2
d
t
.
(
5
)
{\displaystyle s=\int _{\alpha }^{\beta }{\sqrt {1+\left[{\frac {\psi '(t)}{\phi '(t)}}\right]^{2}}}\phi '(t)dt=\int _{\alpha }^{\beta }{\sqrt {[\phi '(t)]^{2}+\left[\psi '(t)\right]^{2}}}dt.\quad (5)}
Kreivės lanko ilgis polinėse koordinatėse .Tegu polinėse koordinatėse kreivės lygtis apibūdinama
ρ
=
f
(
θ
)
,
(
8
)
{\displaystyle \rho =f(\theta ),\quad (8)}
kur
ρ
{\displaystyle \rho }
θ
{\displaystyle \theta }
Užrašysime perėjimo formules iš poliarinių koordinačių į dekarto koordinates:
x
=
ρ
cos
θ
,
y
=
ρ
sin
θ
.
{\displaystyle x=\rho \cos \theta ,\;\;y=\rho \sin \theta .}
Jeigu čia vietoje
ρ
{\displaystyle \rho }
θ
,
{\displaystyle \theta ,}
x
=
f
(
θ
)
cos
θ
,
y
=
f
(
θ
)
sin
θ
.
{\displaystyle x=f(\theta )\cos \theta ,\;\;y=f(\theta )\sin \theta .}
Šias lygtis galima nagrinėti kaip kreivės parametrines lygtis ir kreivės lanko ilgio apskaičiavimui pritaikyti (5) formulę. Tam rasime išvestines nuo x ir y per parametrą
θ
{\displaystyle \theta }
d
x
d
θ
=
f
′
(
θ
)
cos
θ
−
f
(
θ
)
sin
θ
;
d
y
d
θ
=
f
′
(
θ
)
sin
θ
+
f
(
θ
)
cos
θ
.
{\displaystyle {\frac {dx}{d\theta }}=f'(\theta )\cos \theta -f(\theta )\sin \theta ;\;\;{\frac {dy}{d\theta }}=f'(\theta )\sin \theta +f(\theta )\cos \theta .}
Tada
(
d
x
d
θ
)
2
+
(
d
y
d
θ
)
2
=
[
f
′
(
θ
)
]
2
+
[
f
(
θ
)
]
2
=
ρ
′
2
+
ρ
2
.
{\displaystyle \left({\frac {dx}{d\theta }}\right)^{2}+\left({\frac {dy}{d\theta }}\right)^{2}=[f'(\theta )]^{2}+[f(\theta )]^{2}=\rho '^{2}+\rho ^{2}.}
Todėl,
s
=
∫
θ
0
θ
ρ
′
2
+
ρ
2
d
θ
.
{\displaystyle s=\int _{\theta _{0}}^{\theta }{\sqrt {\rho '^{2}+\rho ^{2}}}d\theta .}
Čia patiems mažiausiems (nes matematikai sudaugina ir sudeda mintyse):
(
d
x
d
θ
)
2
=
(
f
′
(
θ
)
cos
θ
−
f
(
θ
)
sin
θ
)
2
=
[
f
′
(
θ
)
]
2
cos
2
θ
−
2
f
′
(
θ
)
cos
θ
f
(
θ
)
sin
θ
+
[
f
(
θ
)
]
2
sin
2
θ
;
{\displaystyle \left({\frac {dx}{d\theta }}\right)^{2}=(f'(\theta )\cos \theta -f(\theta )\sin \theta )^{2}=[f'(\theta )]^{2}\cos ^{2}\theta -2f'(\theta )\cos \theta f(\theta )\sin \theta +[f(\theta )]^{2}\sin ^{2}\theta ;}
(
d
y
d
θ
)
2
=
(
f
′
(
θ
)
sin
θ
+
f
(
θ
)
cos
θ
)
2
=
[
f
′
(
θ
)
]
2
sin
2
θ
+
2
f
′
(
θ
)
sin
θ
f
(
θ
)
cos
θ
+
[
f
(
θ
)
]
2
cos
2
θ
.
{\displaystyle \left({\frac {dy}{d\theta }}\right)^{2}=(f'(\theta )\sin \theta +f(\theta )\cos \theta )^{2}=[f'(\theta )]^{2}\sin ^{2}\theta +2f'(\theta )\sin \theta f(\theta )\cos \theta +[f(\theta )]^{2}\cos ^{2}\theta .}
Baigdami išspręsime pavyzdį, kai
ρ
=
θ
2
,
{\displaystyle \rho =\theta ^{2},}
l
=
∫
L
d
s
=
∫
0
2
π
ρ
2
+
(
ρ
′
)
2
d
θ
=
∫
0
2
π
(
θ
2
)
2
+
(
2
θ
)
2
d
θ
=
∫
0
2
π
θ
4
+
4
θ
2
d
θ
=
{\displaystyle l=\int _{L}ds=\int _{0}^{2\pi }{\sqrt {\rho ^{2}+(\rho ')^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {(\theta ^{2})^{2}+(2\theta )^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {\theta ^{4}+4\theta ^{2}}}d\theta =}
=
∫
0
2
π
θ
θ
2
+
4
d
θ
=
1
3
(
θ
2
+
4
)
3
2
|
0
2
π
=
1
3
(
(
2
π
)
2
+
4
)
3
2
−
1
3
(
0
2
+
4
)
3
2
=
{\displaystyle =\int _{0}^{2\pi }\theta {\sqrt {\theta ^{2}+4}}\;d\theta ={\frac {1}{3}}\left(\theta ^{2}+4\right)^{3 \over 2}|_{0}^{2\pi }={\frac {1}{3}}\left((2\pi )^{2}+4\right)^{3 \over 2}-{\frac {1}{3}}\left(0^{2}+4\right)^{3 \over 2}=}
=
1
3
(
4
(
π
2
+
1
)
)
3
2
−
8
3
=
(
286.6887126
−
8
)
/
3
=
92.896237521771263212813630524448
;
{\displaystyle ={\frac {1}{3}}(4(\pi ^{2}+1))^{3 \over 2}-{\frac {8}{3}}=(286.6887126-8)/3=92.896237521771263212813630524448;}
l
=
∫
L
d
s
=
∫
0
2
π
ρ
2
+
(
ρ
′
)
2
d
θ
=
∫
0
2
π
(
θ
2
)
2
+
(
2
θ
)
2
d
θ
=
∫
0
2
π
θ
4
+
4
θ
2
d
θ
=
{\displaystyle l=\int _{L}ds=\int _{0}^{2\pi }{\sqrt {\rho ^{2}+(\rho ')^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {(\theta ^{2})^{2}+(2\theta )^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {\theta ^{4}+4\theta ^{2}}}d\theta =}
=
∫
0
2
π
θ
θ
2
+
4
d
θ
=
1
2
∫
0
2
π
θ
2
+
4
d
(
θ
2
+
4
)
=
1
2
⋅
(
θ
2
+
4
)
3
/
2
3
2
|
0
2
π
=
(
θ
2
+
4
)
3
/
2
3
|
0
2
π
=
92.89623752
;
{\displaystyle =\int _{0}^{2\pi }\theta {\sqrt {\theta ^{2}+4}}\;d\theta ={\frac {1}{2}}\int _{0}^{2\pi }{\sqrt {\theta ^{2}+4}}\;d(\theta ^{2}+4)={\frac {1}{2}}\cdot {\frac {(\theta ^{2}+4)^{3/2}}{\frac {3}{2}}}|_{0}^{2\pi }={\frac {(\theta ^{2}+4)^{3/2}}{3}}|_{0}^{2\pi }=92.89623752;}
l
=
∫
L
d
s
=
∫
0
2
π
ρ
2
+
(
ρ
′
)
2
d
θ
=
∫
0
2
π
(
θ
2
)
2
+
(
2
θ
)
2
d
θ
=
∫
0
2
π
θ
4
+
4
θ
2
d
θ
=
{\displaystyle l=\int _{L}ds=\int _{0}^{2\pi }{\sqrt {\rho ^{2}+(\rho ')^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {(\theta ^{2})^{2}+(2\theta )^{2}}}d\theta =\int _{0}^{2\pi }{\sqrt {\theta ^{4}+4\theta ^{2}}}d\theta =}
=
∫
0
2
π
θ
θ
2
+
4
d
θ
=
1
2
∫
0
2
π
θ
2
+
4
d
(
θ
2
)
=
1
2
(
2
⋅
4
3
+
2
θ
2
3
)
θ
2
+
4
|
0
2
π
=
{\displaystyle =\int _{0}^{2\pi }\theta {\sqrt {\theta ^{2}+4}}\;d\theta ={\frac {1}{2}}\int _{0}^{2\pi }{\sqrt {\theta ^{2}+4}}\;d(\theta ^{2})={\frac {1}{2}}\left({\frac {2\cdot 4}{3}}+{\frac {2\theta ^{2}}{3}}\right){\sqrt {\theta ^{2}+4}}|_{0}^{2\pi }=}
=
1
2
(
8
3
+
2
⋅
(
2
π
)
2
3
)
(
2
π
)
2
+
4
−
1
2
(
8
3
+
2
⋅
0
2
3
)
0
2
+
4
=
(
4
3
+
4
π
2
3
)
4
π
2
+
4
−
8
3
=
{\displaystyle ={\frac {1}{2}}\left({\frac {8}{3}}+{\frac {2\cdot (2\pi )^{2}}{3}}\right){\sqrt {(2\pi )^{2}+4}}-{\frac {1}{2}}\left({\frac {8}{3}}+{\frac {2\cdot 0^{2}}{3}}\right){\sqrt {0^{2}+4}}=\left({\frac {4}{3}}+{\frac {4\pi ^{2}}{3}}\right){\sqrt {4\pi ^{2}+4}}-{\frac {8}{3}}=}
=
8
+
8
π
2
3
π
2
+
1
−
8
3
=
8
(
π
2
+
1
)
3
π
2
+
1
−
8
3
=
8
(
π
2
+
1
)
3
2
3
−
8
3
=
{\displaystyle ={\frac {8+8\pi ^{2}}{3}}{\sqrt {\pi ^{2}+1}}-{\frac {8}{3}}={\frac {8(\pi ^{2}+1)}{3}}{\sqrt {\pi ^{2}+1}}-{\frac {8}{3}}={\frac {8(\pi ^{2}+1)^{3 \over 2}}{3}}-{\frac {8}{3}}=}
=95,562904188437929879480297191115-2,6(6)=92,896237521771263212813630524448;
čia
d
(
θ
2
+
4
)
=
2
θ
d
θ
,
d
θ
=
d
(
θ
2
+
4
)
2
θ
{\displaystyle d(\theta ^{2}+4)=2\theta \;d\theta ,\;d\theta ={\frac {d(\theta ^{2}+4)}{2\theta }}}
∫
x
x
2
±
a
2
d
x
=
1
3
(
x
2
±
a
2
)
3
2
{\displaystyle \int x{\sqrt {x^{2}\pm a^{2}}}dx={\frac {1}{3}}\left(x^{2}\pm a^{2}\right)^{3 \over 2}}
∫
a
x
+
b
d
x
=
(
2
b
3
a
+
2
x
3
)
a
x
+
b
.
{\displaystyle \int {\sqrt {ax+b}}dx=\left({\frac {2b}{3a}}+{\frac {2x}{3}}\right){\sqrt {ax+b}}.}
Va čia "Free Pascal" kodas: var
a:longint;
c:real;
begin
for a:=1 to 628318531 do
c:=c+0.00000001*sqrt(sqr(sqr(a*0.00000001))+sqr(a*2.0/100000000));
writeln(c);
readln;
end.
kuris duoda atsakymą 92,8962378457359 po 16 sekundžių su 2,6 GHz procesoriumi. Optimizuotas šio kodo variantas: var
a:longint;
c,b:real;
begin
for a:=1 to 628318531 do
c:=c+sqrt(sqr(sqr(a*0.00000001))+sqr(a*0.00000002));
b:=c*0.00000001;
writeln(b);
readln;
end.
duoda atsakymą 92,8962378457489 po 11 sekundžių su 2,6 GHz procesoriumi. Šis kodas: var
a:longint;
c,b:real;
begin
for a:=1 to 62831853 do
c:=c+sqrt(sqr(sqr(a*0.0000001))+sqr((sqr(a*0.0000001)-sqr((a-1)*0.0000001))/0.0000001));
b:=c*0.0000001;
writeln(b);
readln;
end.
duoda atsakymą 92,8962389233553 po dviejų sekundžių. Tikslesnė (ne daug tikslesnė, nes kaip tik
2
π
⋅
10
8
=
628318530.7179586476925286766559
{\displaystyle 2\pi \cdot 10^{8}=628318530.7179586476925286766559}
var
a:longint;
c,b:real;
begin
for a:=1 to 628318531 do
c:=c+sqrt(sqr(sqr(a*0.00000001))+sqr((sqr(a*0.00000001)-sqr((a-1)*0.00000001))*100000000));
b:=c*0.00000001;
writeln(b);
readln;
end.
duoda atsakymą 92,8962378085099 po 15 sekundžių su 2,6 GHz procesoriumi. Labiausiai teoriją atitinkantis kodas yra šis: var
a:longint;
c,b:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(sqr(a*0.0000000062831853))+sqr((sqr(a*0.0000000062831853)-sqr((a-1)*0.0000000062831853))/0.0000000062831853));
b:=c*0.0000000062831853;
writeln(b);
readln;
end.
duodantis atsakymą 92,8962373310520 po 30 sekundžių su 2,6 GHz procesoriumi. Su patikslinta
2
π
{\displaystyle 2\pi }
var
a:longint;
c,b:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(sqr(a*0.000000006283185307179586))+sqr((sqr(a*0.000000006283185307179586)-sqr((a-1)*0.000000006283185307179586))/0.000000006283185307179586));
b:=c*0.000000006283185307179586;
writeln(b);
readln;
end
gauname atsakymą 92,8962376285006 po 31 sekundės su 2,6 GHz procesoriumi. Panaudojus vietoje dalybos daugybą (
1
2
π
10
9
=
159154943.09189533576888376337251
{\displaystyle {\frac {1}{\frac {2\pi }{10^{9}}}}=159154943.09189533576888376337251}
var
a:longint;
c,b:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(sqr(a*0.0000000062831853))+sqr((sqr(a*0.0000000062831853)-sqr((a-1)*0.0000000062831853))*159154943.0918953));
b:=c*0.0000000062831853;
writeln(b);
readln;
end.
gauname atsakymą 92,8962373100457 po 24 sekundžių su 2,6 GHz procesoriumi. Pastebime, kad kodas (dviem kodais aukščiau ir duodantis atsakymą 92,8962378085099), kuris skaičiavo 15 sekundžių turi tokį ryši su šiuo kodu: 24/15=1.6 ir 1000000000/628318531=1.59154943.
Apskaičiuokime kreivės
y
=
x
3
2
,
0
≤
x
≤
4
{\displaystyle y=x^{3 \over 2},\;0\leq x\leq 4}
Randame
y
′
=
3
2
x
1
2
,
1
+
y
′
2
=
1
+
9
4
x
.
{\displaystyle y'={3 \over 2}x^{1 \over 2},\;{\sqrt {1+y'^{2}}}={\sqrt {1+{9 \over 4}x}}.}
L
=
∫
0
4
1
+
9
4
x
d
x
=
4
9
∫
0
4
(
1
+
9
4
x
)
1
2
d
(
1
+
9
4
x
)
=
4
9
⋅
2
3
(
1
+
9
4
x
)
3
2
|
0
4
=
8
27
(
10
10
−
1
)
≈
9
,
0734.
{\displaystyle L=\int _{0}^{4}{\sqrt {1+{9 \over 4}x}}dx={4 \over 9}\int _{0}^{4}(1+{9 \over 4}x)^{1 \over 2}d(1+{9 \over 4}x)={4 \over 9}\cdot {2 \over 3}(1+{9 \over 4}x)^{3 \over 2}|_{0}^{4}={8 \over 27}(10{\sqrt {10}}-1)\approx 9,0734.}
4
3
2
{\displaystyle 4^{3 \over 2}}
c
=
a
2
+
b
2
=
4
2
+
(
4
3
2
)
2
=
16
+
4
3
=
80
≈
8
,
94427.
{\displaystyle c={\sqrt {a^{2}+b^{2}}}={\sqrt {4^{2}+(4^{3 \over 2})^{2}}}={\sqrt {16+4^{3}}}={\sqrt {80}}\approx 8,94427.}
Apskaičiuosime lanko ilgį pusiaukūbinės parabolės
y
=
x
3
/
2
,
{\displaystyle y=x^{3/2},}
0
≤
x
≤
5.
{\displaystyle 0\leq x\leq 5.}
y
=
x
3
/
2
{\displaystyle y=x^{3/2}}
y
′
=
3
2
x
1
2
.
{\displaystyle y'={3 \over 2}x^{1 \over 2}.}
L
=
∫
a
b
1
+
(
f
′
(
x
)
)
2
d
x
=
∫
0
5
1
+
y
′
2
d
x
=
∫
0
5
1
+
9
x
4
d
x
=
4
9
∫
0
5
1
+
9
x
4
d
(
1
+
9
x
4
)
=
{\displaystyle L=\int _{a}^{b}{\sqrt {1+(f'(x))^{2}}}dx=\int _{0}^{5}{\sqrt {1+y'^{2}}}dx=\int _{0}^{5}{\sqrt {1+{9x \over 4}}}dx={4 \over 9}\int _{0}^{5}{\sqrt {1+{9x \over 4}}}d(1+{9x \over 4})=}
=
4
9
(
1
+
9
x
4
)
3
2
3
2
|
0
5
=
8
27
(
1
+
9
x
4
)
3
2
|
0
5
=
8
27
[
(
4
4
+
45
4
)
3
2
−
(
1
+
0
)
3
2
]
=
8
27
[
(
7
2
)
3
−
1
]
=
335
27
≈
12
,
4074
;
{\displaystyle ={{4 \over 9}(1+{9x \over 4})^{3 \over 2} \over {3 \over 2}}|_{0}^{5}={8 \over 27}(1+{9x \over 4})^{3 \over 2}|_{0}^{5}={8 \over 27}[({4 \over 4}+{45 \over 4})^{3 \over 2}-(1+0)^{3 \over 2}]={8 \over 27}[({7 \over 2})^{3}-1]={335 \over 27}\approx 12,4074;}
kur
d
(
1
+
9
x
4
)
=
9
4
d
x
{\displaystyle d(1+{9x \over 4})={9 \over 4}dx}
d
x
=
4
9
d
(
1
+
9
x
4
)
{\displaystyle dx={4 \over 9}d(1+{9x \over 4})}
5
3
2
{\displaystyle 5^{3 \over 2}}
c
=
a
2
+
b
2
=
5
2
+
(
5
3
2
)
2
=
25
+
5
3
=
150
=
12
,
2474487.
{\displaystyle c={\sqrt {a^{2}+b^{2}}}={\sqrt {5^{2}+(5^{3 \over 2})^{2}}}={\sqrt {25+5^{3}}}={\sqrt {150}}=12,2474487.}
Apskaičiuosime lanko ilgį pusiaukūbinės parabolės
y
=
x
3
2
,
{\displaystyle y=x^{3 \over 2},}
1
≤
x
≤
5.
{\displaystyle 1\leq x\leq 5.}
y
=
x
3
/
2
{\displaystyle y=x^{3/2}}
y
′
=
(
x
3
2
)
′
=
3
2
x
1
2
.
{\displaystyle y'=(x^{3 \over 2})'={3 \over 2}x^{1 \over 2}.}
L
=
∫
1
5
1
+
y
′
2
d
x
=
∫
1
5
1
+
(
3
x
2
)
2
d
x
=
∫
1
5
1
+
9
x
4
d
x
=
4
9
∫
1
5
1
+
9
x
4
d
(
1
+
9
x
4
)
=
{\displaystyle L=\int _{1}^{5}{\sqrt {1+y'^{2}}}dx=\int _{1}^{5}{\sqrt {1+({3{\sqrt {x}} \over 2})^{2}}}dx=\int _{1}^{5}{\sqrt {1+{9x \over 4}}}dx={4 \over 9}\int _{1}^{5}{\sqrt {1+{9x \over 4}}}d(1+{9x \over 4})=}
=
4
9
⋅
(
1
+
9
x
4
)
3
2
3
2
|
1
5
=
8
27
(
1
+
9
x
4
)
3
2
|
1
5
=
8
27
[
(
4
4
+
45
4
)
3
2
−
(
1
+
9
4
)
3
2
]
=
8
27
[
(
7
2
)
3
−
(
1
+
2
,
25
)
3
]
=
{\displaystyle ={4 \over 9}\cdot {(1+{9x \over 4})^{3 \over 2} \over {3 \over 2}}|_{1}^{5}={8 \over 27}(1+{9x \over 4})^{3 \over 2}|_{1}^{5}={8 \over 27}[({4 \over 4}+{45 \over 4})^{3 \over 2}-(1+{9 \over 4})^{3 \over 2}]={8 \over 27}[({7 \over 2})^{3}-{\sqrt {(1+2,25)^{3}}}]=}
=
8
27
⋅
343
8
−
8
27
⋅
34
,
328125
≈
12
,
7037037
−
1
,
73600617
≈
10
,
96769753
;
{\displaystyle ={8 \over 27}\cdot {343 \over 8}-{8 \over 27}\cdot {\sqrt {34,328125}}\approx 12,7037037-1,73600617\approx 10,96769753;}
kur
d
(
1
+
9
x
4
)
=
9
4
d
x
{\displaystyle d(1+{9x \over 4})={9 \over 4}dx}
d
x
=
4
9
d
(
1
+
9
x
4
)
{\displaystyle dx={4 \over 9}d(1+{9x \over 4})}
5
3
/
2
{\displaystyle 5^{3/2}}
c
=
(
5
−
1
)
2
+
(
5
3
/
2
−
1
)
2
=
16
+
(
125
−
1
)
2
=
16
+
10
,
18033989
2
=
119
,
6393202
=
{\displaystyle c={\sqrt {(5-1)^{2}+(5^{3/2}-1)^{2}}}={\sqrt {16+({\sqrt {125}}-1)^{2}}}={\sqrt {16+10,18033989^{2}}}={\sqrt {119,6393202}}=}
=
10
,
093797606.
{\displaystyle =10,093797606.}
Apskaičiuosime parabolės
y
=
x
2
{\displaystyle y=x^{2}}
0
≤
x
≤
5.
{\displaystyle 0\leq x\leq 5.}
Randame
y
′
=
(
x
2
)
′
=
2
x
{\displaystyle y'=(x^{2})'=2x}
L
=
∫
0
5
1
+
(
2
x
)
2
d
x
=
∫
0
5
1
+
4
x
2
d
x
=
1
4
(
2
x
4
x
2
+
1
+
arsinh
(
2
x
)
)
|
0
5
=
{\displaystyle L=\int _{0}^{5}{\sqrt {1+(2x)^{2}}}dx=\int _{0}^{5}{\sqrt {1+4x^{2}}}dx={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\operatorname {arsinh} (2x))|_{0}^{5}=}
=
1
4
(
2
⋅
5
4
⋅
5
2
+
1
+
arsinh
(
2
⋅
5
)
)
−
1
4
(
2
⋅
0
⋅
4
⋅
0
2
+
1
+
arsinh
(
2
⋅
0
)
)
=
{\displaystyle ={1 \over 4}(2\cdot 5{\sqrt {4\cdot 5^{2}+1}}+\operatorname {arsinh} (2\cdot 5))-{1 \over 4}(2\cdot 0\cdot {\sqrt {4\cdot 0^{2}+1}}+\operatorname {arsinh} (2\cdot 0))=}
=
1
4
(
10
4
⋅
25
+
1
+
arsinh
(
10
)
)
−
1
4
⋅
(
0
+
0
)
=
1
4
(
10
101
+
ln
(
10
+
10
2
+
1
)
)
−
0
=
{\displaystyle ={1 \over 4}(10{\sqrt {4\cdot 25+1}}+\operatorname {arsinh} (10))-{1 \over 4}\cdot (0+0)={1 \over 4}(10{\sqrt {101}}+\ln \left(10+{\sqrt {10^{2}+1}}\right))-0=}
=
1
4
(
10
101
+
ln
(
10
+
101
)
)
=
{\displaystyle ={\frac {1}{4}}(10{\sqrt {101}}+\ln(10+{\sqrt {101}}))=}
=
1
4
(
10
⋅
10.4987562
+
ln
(
20
,
04987562
)
)
=
{\displaystyle ={1 \over 4}(10\cdot 10.4987562+\ln \left(20,04987562\right))=}
=
1
4
(
100.498756
+
2.99822295
)
=
103.4969792
4
=
25.87424479
,
{\displaystyle ={1 \over 4}(100.498756+2.99822295)={\frac {103.4969792}{4}}=25.87424479,}
čia
arsinh
x
=
ln
(
x
+
x
2
+
1
)
.
{\displaystyle \operatorname {arsinh} \,x=\ln \left(x+{\sqrt {x^{2}+1}}\right).}
ln
(
x
+
x
2
+
1
)
=
ln
(
10
+
10
2
+
1
)
=
ln
(
10
+
101
)
=
ln
(
10
+
10.04987562
)
=
2.99822295.
{\displaystyle \ln \left(x+{\sqrt {x^{2}+1}}\right)=\ln \left(10+{\sqrt {10^{2}+1}}\right)=\ln \left(10+{\sqrt {101}}\right)=\ln \left(10+10.04987562\right)=2.99822295.}
Iš kompiuterio kalkuliatoriaus reikšmės (hiperbolinio arksinuso):
arsinh
10
=
2
,
9982229502979697388465955375965
;
{\displaystyle \operatorname {arsinh} \,10=2,9982229502979697388465955375965;}
arsinh
0
=
0.
{\displaystyle \operatorname {arsinh} \,0=0.}
Palyginimui, linijos ilgis nuo taško (0; 0) iki taško (5; 25) yra:
c
=
a
2
+
b
2
=
5
2
+
25
2
=
25
+
625
=
650
=
25.49509757.
{\displaystyle c={\sqrt {a^{2}+b^{2}}}={\sqrt {5^{2}+25^{2}}}={\sqrt {25+625}}={\sqrt {650}}=25.49509757.}
Apskaičiuosime parabolės
y
=
x
2
{\displaystyle y=x^{2}}
1
≤
x
≤
5.
{\displaystyle 1\leq x\leq 5.}
Randame
y
′
=
(
x
2
)
′
=
2
x
{\displaystyle y'=(x^{2})'=2x}
L
=
∫
1
5
1
+
(
2
x
)
2
d
x
=
∫
1
5
1
+
4
x
2
d
x
=
1
4
(
2
x
4
x
2
+
1
+
sinh
−
1
(
2
x
)
)
|
1
5
=
{\displaystyle L=\int _{1}^{5}{\sqrt {1+(2x)^{2}}}dx=\int _{1}^{5}{\sqrt {1+4x^{2}}}dx={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\sinh ^{-1}(2x))|_{1}^{5}=}
=
1
4
(
2
x
4
x
2
+
1
+
arsinh
(
2
x
)
)
|
1
5
=
{\displaystyle ={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\operatorname {arsinh} \,(2x))|_{1}^{5}=}
=
1
4
(
10
4
⋅
25
+
1
+
arsinh
(
10
)
)
−
1
4
(
2
4
⋅
1
+
1
+
arsinh
(
2
)
)
=
{\displaystyle ={1 \over 4}(10{\sqrt {4\cdot 25+1}}+\operatorname {arsinh} \,(10))-{1 \over 4}(2{\sqrt {4\cdot 1+1}}+\operatorname {arsinh} \,(2))=}
=
1
4
(
10
101
+
ln
(
10
+
10
2
+
1
)
)
−
1
4
(
2
5
+
ln
(
2
+
2
2
+
1
)
)
=
{\displaystyle ={1 \over 4}(10{\sqrt {101}}+\ln \left(10+{\sqrt {10^{2}+1}}\right))-{1 \over 4}(2{\sqrt {5}}+\ln \left(2+{\sqrt {2^{2}+1}}\right))=}
=
1
4
(
10
101
+
ln
(
10
+
101
)
)
−
1
4
(
2
5
+
ln
(
2
+
5
)
)
≈
{\displaystyle ={1 \over 4}(10{\sqrt {101}}+\ln \left(10+{\sqrt {101}}\right))-{1 \over 4}(2{\sqrt {5}}+\ln \left(2+{\sqrt {5}}\right))\approx }
≈
1
4
(
100
,
4987562
+
ln
(
20
,
04987562
)
)
−
1
4
(
4
,
472135955
+
ln
(
4
,
236067978
)
)
≈
{\displaystyle \approx {1 \over 4}(100,4987562+\ln \left(20,04987562\right))-{1 \over 4}(4,472135955+\ln \left(4,236067978\right))\approx }
≈
1
4
(
100
,
4987562
+
2.99822295
)
−
1
4
(
4
,
472135955
+
1.44363547
)
=
{\displaystyle \approx {1 \over 4}(100,4987562+2.99822295)-{1 \over 4}(4,472135955+1.44363547)=}
=
25
,
87424479
−
1
,
478942858
=
24.39530193
,
{\displaystyle =25,87424479-1,478942858=24.39530193,}
čia
arsinh
x
=
ln
(
x
+
x
2
+
1
)
.
{\displaystyle \operatorname {arsinh} \,x=\ln \left(x+{\sqrt {x^{2}+1}}\right).}
Iš kompiuterio kalkuliatoriaus reikšmės (hiperbolinio arksinuso):
arsinh
10
=
2
,
9982229502979697388465955375965
;
{\displaystyle \operatorname {arsinh} \,10=2,9982229502979697388465955375965;}
arsinh
2
=
1
,
44363547517881.
{\displaystyle \operatorname {arsinh} \,2=1,44363547517881.}
Palyginimui, tiesės ilgis nuo taško (1; 1) iki taško (5; 25) yra:
c
=
a
2
+
b
2
=
(
5
−
1
)
2
+
(
25
−
1
)
2
=
4
2
+
24
2
=
16
+
576
=
592
=
24.33105012.
{\displaystyle c={\sqrt {a^{2}+b^{2}}}={\sqrt {(5-1)^{2}+(25-1)^{2}}}={\sqrt {4^{2}+24^{2}}}={\sqrt {16+576}}={\sqrt {592}}=24.33105012.}
Wolfram Research integratorius, kad
∫
1
+
4
x
2
d
x
=
1
4
(
2
x
4
x
2
+
1
+
sinh
−
1
(
2
x
)
)
{\displaystyle \int {\sqrt {1+4x^{2}}}dx={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\sinh ^{-1}(2x))}
http://integrals.wolfram.com/index.jsp?expr=%281%2B4x%5E2%29%5E%281%2F2%29&random=false .
Toks būdas neteisingas:
L
=
∫
1
5
1
+
(
2
x
)
2
d
x
=
∫
1
5
1
+
4
x
2
d
x
=
1
4
(
2
x
4
x
2
+
1
+
sinh
−
1
(
2
x
)
)
|
1
5
=
{\displaystyle L=\int _{1}^{5}{\sqrt {1+(2x)^{2}}}dx=\int _{1}^{5}{\sqrt {1+4x^{2}}}dx={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\sinh ^{-1}(2x))|_{1}^{5}=}
=
1
4
(
2
x
4
x
2
+
1
+
(
e
2
x
−
e
−
2
x
2
)
−
1
)
|
1
5
=
1
4
(
2
x
4
x
2
+
1
+
2
e
2
x
−
e
−
2
x
)
|
1
5
=
{\displaystyle ={1 \over 4}(2x{\sqrt {4x^{2}+1}}+({e^{2x}-e^{-2x} \over 2})^{-1})|_{1}^{5}={1 \over 4}(2x{\sqrt {4x^{2}+1}}+{2 \over e^{2x}-e^{-2x}})|_{1}^{5}=}
=
1
4
(
10
100
+
1
+
2
e
10
−
e
−
10
)
−
1
4
(
2
4
+
1
+
2
e
2
−
e
−
2
)
≈
{\displaystyle ={1 \over 4}(10{\sqrt {100+1}}+{2 \over e^{10}-e^{-10}})-{1 \over 4}(2{\sqrt {4+1}}+{2 \over e^{2}-e^{-2}})\approx }
≈
25
,
12471175
−
1
4
(
4
,
472135955
+
2
7
,
389056099
−
0
,
135335283
)
≈
{\displaystyle \approx 25,12471175-{1 \over 4}(4,472135955+{2 \over 7,389056099-0,135335283})\approx }
≈
25
,
12471175
−
1
4
(
4
,
472135955
+
2
7
,
253720816
)
≈
{\displaystyle \approx 25,12471175-{1 \over 4}(4,472135955+{2 \over 7,253720816})\approx }
≈
25
,
12471175
−
1
4
(
4
,
472135955
+
0
,
275720564
)
≈
{\displaystyle \approx 25,12471175-{1 \over 4}(4,472135955+0,275720564)\approx }
≈
25
,
12471175
−
1
,
18696413
≈
23
,
93774762.
{\displaystyle \approx 25,12471175-1,18696413\approx 23,93774762.}
Patikriname atsakymą kitu budu:
L
=
∫
1
5
1
+
y
′
2
d
x
=
∫
1
5
1
+
(
2
x
)
2
d
x
=
∫
1
5
1
+
4
x
2
d
x
=
4
∫
1
5
1
4
+
x
2
d
x
=
{\displaystyle L=\int _{1}^{5}{\sqrt {1+y'^{2}}}dx=\int _{1}^{5}{\sqrt {1+(2x)^{2}}}dx=\int _{1}^{5}{\sqrt {1+4x^{2}}}dx={\sqrt {4}}\int _{1}^{5}{\sqrt {{1 \over 4}+x^{2}}}dx=}
=
2
(
x
2
1
4
+
x
2
+
1
4
2
ln
|
x
+
x
2
+
1
4
|
)
|
1
5
=
{\displaystyle =2({x \over 2}{\sqrt {{1 \over 4}+x^{2}}}+{\frac {1 \over 4}{2}}\ln \left|x+{\sqrt {x^{2}+{1 \over 4}}}\right|)|_{1}^{5}=}
=
2
(
5
2
0
,
25
+
5
2
+
0
,
25
2
ln
|
5
+
5
2
+
0
,
25
|
)
−
2
(
1
2
0
,
25
+
1
2
+
0
,
25
2
ln
|
1
+
1
2
+
0
,
25
|
)
=
{\displaystyle =2({5 \over 2}{\sqrt {0,25+5^{2}}}+{\frac {0,25}{2}}\ln \left|5+{\sqrt {5^{2}+0,25}}\right|)-2({1 \over 2}{\sqrt {0,25+1^{2}}}+{\frac {0,25}{2}}\ln \left|1+{\sqrt {1^{2}+0,25}}\right|)=}
=
2
(
2
,
5
25
,
25
+
0
,
125
ln
|
5
+
25
,
25
|
)
−
2
(
1
2
1
,
25
+
0
,
125
ln
|
1
+
1
,
25
|
)
≈
{\displaystyle =2(2,5{\sqrt {25,25}}+0,125\ln \left|5+{\sqrt {25,25}}\right|)-2({1 \over 2}{\sqrt {1,25}}+0,125\ln \left|1+{\sqrt {1,25}}\right|)\approx }
≈
2
(
2
,
5
⋅
5
,
024937811
+
0
,
125
ln
|
5
+
5
,
024937811
|
)
−
2
(
1
,
118033989
2
+
0
,
125
⋅
ln
|
1
+
1
,
118033989
|
)
≈
{\displaystyle \approx 2(2,5\cdot 5,024937811+0,125\ln |5+5,024937811|)-2({1,118033989 \over 2}+0,125\cdot \ln |1+1,118033989|)\approx }
≈
2
(
12
,
56234453
+
0
,
125
⋅
2
,
30507577
)
−
2
(
0
,
559016994
+
0
,
125
⋅
0
,
750488294
)
=
{\displaystyle \approx 2(12,56234453+0,125\cdot 2,30507577)-2(0,559016994+0,125\cdot 0,750488294)=}
=
2
(
12
,
56234453
+
0
,
288134471
)
−
2
(
0
,
559016994
+
0
,
093811036
)
=
25
,
700958
−
1
,
30565606
=
24
,
39530194.
{\displaystyle =2(12,56234453+0,288134471)-2(0,559016994+0,093811036)=25,700958-1,30565606=24,39530194.}
Apskaičiuosime parabolės
y
=
x
2
{\displaystyle y=x^{2}}
0
≤
x
≤
4.
{\displaystyle 0\leq x\leq 4.}
Randame
y
′
=
(
x
2
)
′
=
2
x
{\displaystyle y'=(x^{2})'=2x}
L
=
∫
0
4
1
+
(
2
x
)
2
d
x
=
∫
0
4
1
+
4
x
2
d
x
=
1
4
(
2
x
4
x
2
+
1
+
arsinh
(
2
x
)
)
|
0
4
=
{\displaystyle L=\int _{0}^{4}{\sqrt {1+(2x)^{2}}}dx=\int _{0}^{4}{\sqrt {1+4x^{2}}}dx={1 \over 4}(2x{\sqrt {4x^{2}+1}}+\operatorname {arsinh} (2x))|_{0}^{4}=}
=
1
4
(
2
⋅
4
4
⋅
4
2
+
1
+
arsinh
(
2
⋅
4
)
)
−
1
4
(
2
⋅
0
⋅
4
⋅
0
2
+
1
+
arsinh
(
2
⋅
0
)
)
=
{\displaystyle ={1 \over 4}(2\cdot 4{\sqrt {4\cdot 4^{2}+1}}+\operatorname {arsinh} (2\cdot 4))-{1 \over 4}(2\cdot 0\cdot {\sqrt {4\cdot 0^{2}+1}}+\operatorname {arsinh} (2\cdot 0))=}
=
1
4
(
8
4
⋅
16
+
1
+
arsinh
(
8
)
)
−
1
4
⋅
(
0
+
0
)
=
1
4
(
8
64
+
1
+
ln
(
8
+
8
2
+
1
)
)
−
0
=
{\displaystyle ={1 \over 4}(8{\sqrt {4\cdot 16+1}}+\operatorname {arsinh} (8))-{1 \over 4}\cdot (0+0)={1 \over 4}(8{\sqrt {64+1}}+\ln \left(8+{\sqrt {8^{2}+1}}\right))-0=}
=
1
4
(
8
65
+
ln
(
8
+
65
)
)
=
1
4
(
8
⋅
8.062257748
+
ln
(
8
+
8.062257748
)
)
=
{\displaystyle ={1 \over 4}(8{\sqrt {65}}+\ln \left(8+{\sqrt {65}}\right))={1 \over 4}(8\cdot 8.062257748+\ln \left(8+8.062257748\right))=}
=
1
4
(
64.49806199
+
ln
(
16.06225775
)
)
=
1
4
(
64.49806199
+
2.776472281
)
=
67.27453427
4
=
16.81863357
,
{\displaystyle ={1 \over 4}(64.49806199+\ln(16.06225775))={1 \over 4}(64.49806199+2.776472281)={\frac {67.27453427}{4}}=16.81863357,}
čia
arsinh
x
=
ln
(
x
+
x
2
+
1
)
.
{\displaystyle \operatorname {arsinh} \,x=\ln \left(x+{\sqrt {x^{2}+1}}\right).}
ln
(
x
+
x
2
+
1
)
=
ln
(
8
+
8
2
+
1
)
=
ln
(
8
+
65
)
=
ln
(
8
+
8.062257748
)
=
ln
(
16.06225775
)
=
2.776472281.
{\displaystyle \ln(x+{\sqrt {x^{2}+1}})=\ln(8+{\sqrt {8^{2}+1}})=\ln(8+{\sqrt {65}})=\ln(8+8.062257748)=\ln(16.06225775)=2.776472281.}
Iš kompiuterio kalkuliatoriaus reikšmės (hiperbolinio arksinuso):
arsinh
8
=
2
,
7764722807237176735308040270285
;
{\displaystyle \operatorname {arsinh} \,8=2,7764722807237176735308040270285;}
arsinh
0
=
0.
{\displaystyle \operatorname {arsinh} \,0=0.}
Apskaičiuosime parabolės
y
=
x
2
{\displaystyle y=x^{2}}
0
≤
x
≤
4.
{\displaystyle 0\leq x\leq 4.}
Randame
y
′
=
(
x
2
)
′
=
2
x
{\displaystyle y'=(x^{2})'=2x}
integralų lentele
∫
x
2
+
a
d
x
=
x
2
a
+
x
2
+
a
2
ln
|
x
+
x
2
+
a
|
{\displaystyle \int {\sqrt {x^{2}+a}}\;{\mathsf {d}}x={\frac {x}{2}}{\sqrt {a+x^{2}}}+{\frac {a}{2}}\ln \left|x+{\sqrt {x^{2}+a}}\right|}
L
=
∫
0
4
1
+
y
′
2
d
x
=
∫
0
4
1
+
(
2
x
)
2
d
x
=
∫
0
4
1
+
4
x
2
d
x
=
4
∫
0
4
1
4
+
x
2
d
x
=
{\displaystyle L=\int _{0}^{4}{\sqrt {1+y'^{2}}}dx=\int _{0}^{4}{\sqrt {1+(2x)^{2}}}dx=\int _{0}^{4}{\sqrt {1+4x^{2}}}dx={\sqrt {4}}\int _{0}^{4}{\sqrt {{1 \over 4}+x^{2}}}dx=}
=
2
(
x
2
0
,
25
+
x
2
+
0
,
25
2
ln
|
x
+
x
2
+
0
,
25
|
)
|
0
4
=
{\displaystyle =2({x \over 2}{\sqrt {0,25+x^{2}}}+{\frac {0,25}{2}}\ln \left|x+{\sqrt {x^{2}+0,25}}\right|)|_{0}^{4}=}
=
2
(
4
2
0
,
25
+
4
2
+
0
,
25
2
ln
|
4
+
4
2
+
0
,
25
|
)
−
2
(
0
2
0
,
25
+
0
2
+
0
,
25
2
ln
|
0
+
0
2
+
0
,
25
|
)
=
{\displaystyle =2({4 \over 2}{\sqrt {0,25+4^{2}}}+{\frac {0,25}{2}}\ln \left|4+{\sqrt {4^{2}+0,25}}\right|)-2({0 \over 2}{\sqrt {0,25+0^{2}}}+{\frac {0,25}{2}}\ln \left|0+{\sqrt {0^{2}+0,25}}\right|)=}
=
2
(
2
16
,
25
+
0
,
125
ln
|
4
+
16
,
25
|
)
−
2
(
0
+
0
,
125
ln
|
0
+
0
,
25
|
)
=
{\displaystyle =2(2{\sqrt {16,25}}+0,125\ln \left|4+{\sqrt {16,25}}\right|)-2(0+0,125\ln \left|0+{\sqrt {0,25}}\right|)=}
≈
2
(
2
⋅
4
,
031128874
+
0
,
125
ln
|
8
,
031128874
|
)
−
2
(
0
,
125
⋅
ln
|
0
,
5
|
)
≈
{\displaystyle \approx 2(2\cdot 4,031128874+0,125\ln |8,031128874|)-2(0,125\cdot \ln |0,5|)\approx }
≈
2
(
8
,
062257748
+
0
,
125
⋅
2
,
0833251
)
−
2
(
0
,
125
⋅
(
−
0
,
69314718
)
)
=
{\displaystyle \approx 2(8,062257748+0,125\cdot 2,0833251)-2(0,125\cdot (-0,69314718))=}
=
2
(
8
,
062257748
+
0
,
260415637
)
−
2
(
−
0
,
086643397
)
=
16
,
64534677
+
0
,
173286795
=
16
,
81863357.
{\displaystyle =2(8,062257748+0,260415637)-2(-0,086643397)=16,64534677+0,173286795=16,81863357.}
Palyginimui, tiesios linijos ilgis nuo taško (0; 0) iki taško (4; 16) yra
c
=
x
2
+
y
2
=
4
2
+
16
2
=
272
=
16
,
4924225.
{\displaystyle c={\sqrt {x^{2}+y^{2}}}={\sqrt {4^{2}+16^{2}}}={\sqrt {272}}=16,4924225.}
Patikrinsime parbolės lanko ilgį padalindami parabolės šaką į 10 atkarpų-tiesių, kai 0<x<4. Kiekvienos atkarpos projekcijos į Ox ašį ilgis yra 0,4. Todėl reikia gauti visas x reikšmes:
x
0
=
0
;
{\displaystyle x_{0}=0;}
x
1
=
0.4
;
{\displaystyle x_{1}=0.4;}
x
2
=
2
⋅
0.4
=
0.8
;
{\displaystyle x_{2}=2\cdot 0.4=0.8;}
x
3
=
3
⋅
0.4
=
1.2
;
{\displaystyle x_{3}=3\cdot 0.4=1.2;}
x
4
=
4
⋅
0.4
=
1.6
;
{\displaystyle x_{4}=4\cdot 0.4=1.6;}
x
5
=
5
⋅
0.4
=
2
;
{\displaystyle x_{5}=5\cdot 0.4=2;}
x
6
=
6
⋅
0.4
=
2.4
;
{\displaystyle x_{6}=6\cdot 0.4=2.4;}
x
7
=
7
⋅
0.4
=
2.8
;
{\displaystyle x_{7}=7\cdot 0.4=2.8;}
x
8
=
8
⋅
0.4
=
3.2
;
{\displaystyle x_{8}=8\cdot 0.4=3.2;}
x
9
=
9
⋅
0.4
=
3.6
;
{\displaystyle x_{9}=9\cdot 0.4=3.6;}
x
10
=
10
⋅
0.4
=
4.
{\displaystyle x_{10}=10\cdot 0.4=4.}
Dabar toliau reikia surasti visas y reikšmes, įstačius x reikšmes:
y
0
=
x
0
2
=
0
2
=
0
;
{\displaystyle y_{0}=x_{0}^{2}=0^{2}=0;}
y
1
=
x
1
2
=
0.4
2
=
0.16
;
{\displaystyle y_{1}=x_{1}^{2}=0.4^{2}=0.16;}
y
2
=
x
2
2
=
0.8
2
=
0.64
;
{\displaystyle y_{2}=x_{2}^{2}=0.8^{2}=0.64;}
y
3
=
x
3
2
=
1.2
2
=
1.44
;
{\displaystyle y_{3}=x_{3}^{2}=1.2^{2}=1.44;}
y
4
=
x
4
2
=
1.6
2
=
2.56
;
{\displaystyle y_{4}=x_{4}^{2}=1.6^{2}=2.56;}
y
5
=
x
5
2
=
2
2
=
4
;
{\displaystyle y_{5}=x_{5}^{2}=2^{2}=4;}
y
6
=
x
6
2
=
2.4
2
=
5.76
;
{\displaystyle y_{6}=x_{6}^{2}=2.4^{2}=5.76;}
y
7
=
x
7
2
=
2.8
2
=
7.84
;
{\displaystyle y_{7}=x_{7}^{2}=2.8^{2}=7.84;}
y
8
=
x
8
2
=
3.2
2
=
10.24
;
{\displaystyle y_{8}=x_{8}^{2}=3.2^{2}=10.24;}
y
9
=
x
9
2
=
3.6
2
=
12.96
;
{\displaystyle y_{9}=x_{9}^{2}=3.6^{2}=12.96;}
y
10
=
x
10
2
=
4
2
=
16.
{\displaystyle y_{10}=x_{10}^{2}=4^{2}=16.}
Dabar belieka surasti atkarpu ilgius kaip nuo taško (0; 0) iki taško (0,4; 0,16); nuo taško (0,4; 0,16) iki (0,8; 0,64) ir taip toliau:
a
1
=
(
x
1
−
x
0
)
2
+
(
y
1
−
y
0
)
2
=
(
0.4
−
0
)
2
+
(
0.16
−
0
)
2
=
0.16
+
0.0256
=
0.1856
=
0.430813184
;
{\displaystyle a_{1}={\sqrt {(x_{1}-x_{0})^{2}+(y_{1}-y_{0})^{2}}}={\sqrt {(0.4-0)^{2}+(0.16-0)^{2}}}={\sqrt {0.16+0.0256}}={\sqrt {0.1856}}=0.430813184;}
a
2
=
(
x
2
−
x
1
)
2
+
(
y
2
−
y
1
)
2
=
(
0.8
−
0.4
)
2
+
(
0.64
−
0.16
)
2
=
0.16
+
0.2304
=
0.3904
=
0.624819974
;
{\displaystyle a_{2}={\sqrt {(x_{2}-x_{1})^{2}+(y_{2}-y_{1})^{2}}}={\sqrt {(0.8-0.4)^{2}+(0.64-0.16)^{2}}}={\sqrt {0.16+0.2304}}={\sqrt {0.3904}}=0.624819974;}
a
3
=
(
x
3
−
x
2
)
2
+
(
y
3
−
y
2
)
2
=
(
1.2
−
0.8
)
2
+
(
1.44
−
0.64
)
2
=
0.16
+
0.64
=
0.8
=
0.894427191
;
{\displaystyle a_{3}={\sqrt {(x_{3}-x_{2})^{2}+(y_{3}-y_{2})^{2}}}={\sqrt {(1.2-0.8)^{2}+(1.44-0.64)^{2}}}={\sqrt {0.16+0.64}}={\sqrt {0.8}}=0.894427191;}
a
4
=
(
x
4
−
x
3
)
2
+
(
y
4
−
y
3
)
2
=
(
1.6
−
1.2
)
2
+
(
2.56
−
1.44
)
2
=
0.16
+
1.2544
=
1.4144
=
1.1892855
;
{\displaystyle a_{4}={\sqrt {(x_{4}-x_{3})^{2}+(y_{4}-y_{3})^{2}}}={\sqrt {(1.6-1.2)^{2}+(2.56-1.44)^{2}}}={\sqrt {0.16+1.2544}}={\sqrt {1.4144}}=1.1892855;}
a
5
=
(
x
5
−
x
4
)
2
+
(
y
5
−
y
4
)
2
=
(
2
−
1.6
)
2
+
(
4
−
2.56
)
2
=
0.16
+
2.0736
=
2.2336
=
1.494523335
;
{\displaystyle a_{5}={\sqrt {(x_{5}-x_{4})^{2}+(y_{5}-y_{4})^{2}}}={\sqrt {(2-1.6)^{2}+(4-2.56)^{2}}}={\sqrt {0.16+2.0736}}={\sqrt {2.2336}}=1.494523335;}
a
6
=
(
x
6
−
x
5
)
2
+
(
y
6
−
y
5
)
2
=
(
2.4
−
2
)
2
+
(
5.76
−
4
)
2
=
0.16
+
3.0976
=
3.2576
=
1.804882268
;
{\displaystyle a_{6}={\sqrt {(x_{6}-x_{5})^{2}+(y_{6}-y_{5})^{2}}}={\sqrt {(2.4-2)^{2}+(5.76-4)^{2}}}={\sqrt {0.16+3.0976}}={\sqrt {3.2576}}=1.804882268;}
a
7
=
(
x
7
−
x
6
)
2
+
(
y
7
−
y
6
)
2
=
(
2.8
−
2.4
)
2
+
(
7.84
−
5.76
)
2
=
0.16
+
4.3264
=
4.4864
=
2.118112367
;
{\displaystyle a_{7}={\sqrt {(x_{7}-x_{6})^{2}+(y_{7}-y_{6})^{2}}}={\sqrt {(2.8-2.4)^{2}+(7.84-5.76)^{2}}}={\sqrt {0.16+4.3264}}={\sqrt {4.4864}}=2.118112367;}
a
8
=
(
x
8
−
x
7
)
2
+
(
y
8
−
y
7
)
2
=
(
3.2
−
2.8
)
2
+
(
10.24
−
7.84
)
2
=
0.16
+
5.76
=
5.92
=
2.433105012
;
{\displaystyle a_{8}={\sqrt {(x_{8}-x_{7})^{2}+(y_{8}-y_{7})^{2}}}={\sqrt {(3.2-2.8)^{2}+(10.24-7.84)^{2}}}={\sqrt {0.16+5.76}}={\sqrt {5.92}}=2.433105012;}
a
9
=
(
x
9
−
x
8
)
2
+
(
y
9
−
y
8
)
2
=
(
3.6
−
3.2
)
2
+
(
12.96
−
10.24
)
2
=
0.16
+
7.3984
=
7.5584
=
2.749254444
;
{\displaystyle a_{9}={\sqrt {(x_{9}-x_{8})^{2}+(y_{9}-y_{8})^{2}}}={\sqrt {(3.6-3.2)^{2}+(12.96-10.24)^{2}}}={\sqrt {0.16+7.3984}}={\sqrt {7.5584}}=2.749254444;}
a
10
=
(
x
10
−
x
9
)
2
+
(
y
10
−
y
9
)
2
=
(
4
−
3.6
)
2
+
(
16
−
12.96
)
2
=
0.16
+
9.2416
=
9.4016
=
3.066202863.
{\displaystyle a_{10}={\sqrt {(x_{10}-x_{9})^{2}+(y_{10}-y_{9})^{2}}}={\sqrt {(4-3.6)^{2}+(16-12.96)^{2}}}={\sqrt {0.16+9.2416}}={\sqrt {9.4016}}=3.066202863.}
Toliau reikia sudėti visų atkarpų ilgį, kad gauti parabolės šakos ilgį, kai x kinta nuo 0 iki 4. Gauname:
L
=
a
1
+
a
2
+
a
3
+
a
4
+
a
5
+
a
6
+
a
7
+
a
8
+
a
9
+
a
10
=
{\displaystyle L=a_{1}+a_{2}+a_{3}+a_{4}+a_{5}+a_{6}+a_{7}+a_{8}+a_{9}+a_{10}=}
=
0.430813184
+
0.624819974
+
0.894427191
+
1.1892855
+
1.494523335
+
1.804882268
+
2.118112367
+
2.433105012
+
2.749254444
+
3.066202863
=
16.80542614.
{\displaystyle =0.430813184+0.624819974+0.894427191+1.1892855+1.494523335+1.804882268+2.118112367+2.433105012+2.749254444+3.066202863=16.80542614.}
Padalinus į daugiau dalių atsakymas taptų panašesnis į atsakymą gautą integravimo budu.
Apskaičiuosime parabolės
y
=
x
2
{\displaystyle y=x^{2}}
1
≤
x
≤
4.
{\displaystyle 1\leq x\leq 4.}
Randame
y
′
=
(
x
2
)
′
=
2
x
{\displaystyle y'=(x^{2})'=2x}
integralų lentele gauname
L
=
∫
1
4
1
+
y
′
2
d
x
=
∫
1
4
1
+
(
2
x
)
2
d
x
=
∫
1
4
1
+
4
x
2
d
x
=
4
∫
1
4
1
4
+
x
2
d
x
=
{\displaystyle L=\int _{1}^{4}{\sqrt {1+y'^{2}}}dx=\int _{1}^{4}{\sqrt {1+(2x)^{2}}}dx=\int _{1}^{4}{\sqrt {1+4x^{2}}}dx={\sqrt {4}}\int _{1}^{4}{\sqrt {{1 \over 4}+x^{2}}}dx=}
=
2
(
x
2
0
,
25
+
x
2
+
0
,
25
2
ln
|
x
+
x
2
+
0
,
25
|
)
|
1
4
=
{\displaystyle =2({x \over 2}{\sqrt {0,25+x^{2}}}+{\frac {0,25}{2}}\ln \left|x+{\sqrt {x^{2}+0,25}}\right|)|_{1}^{4}=}
=
2
(
4
2
0
,
25
+
4
2
+
0
,
25
2
ln
|
4
+
4
2
+
0
,
25
|
)
−
2
(
1
2
0
,
25
+
1
2
+
0
,
25
2
ln
|
1
+
1
2
+
0
,
25
|
)
=
{\displaystyle =2({4 \over 2}{\sqrt {0,25+4^{2}}}+{\frac {0,25}{2}}\ln \left|4+{\sqrt {4^{2}+0,25}}\right|)-2({1 \over 2}{\sqrt {0,25+1^{2}}}+{\frac {0,25}{2}}\ln \left|1+{\sqrt {1^{2}+0,25}}\right|)=}
=
2
(
2
16
,
25
+
0
,
125
ln
|
4
+
16
,
25
|
)
−
2
(
1
2
1
,
25
+
0
,
125
ln
|
1
+
1
,
25
|
)
=
{\displaystyle =2(2{\sqrt {16,25}}+0,125\ln \left|4+{\sqrt {16,25}}\right|)-2({1 \over 2}{\sqrt {1,25}}+0,125\ln \left|1+{\sqrt {1,25}}\right|)=}
≈
2
(
2
⋅
4
,
031128874
+
0
,
125
ln
|
8
,
031128874
|
)
−
(
1
,
118033989
+
0
,
25
⋅
ln
|
1
+
1
,
118033989
|
)
≈
{\displaystyle \approx 2(2\cdot 4,031128874+0,125\ln \left|8,031128874\right|)-(1,118033989+0,25\cdot \ln \left|1+1,118033989\right|)\approx }
≈
2
(
8
,
062257748
+
0
,
125
⋅
2
,
0833251
)
−
(
1
,
118033989
+
0
,
25
⋅
0
,
750488294
)
=
{\displaystyle \approx 2(8,062257748+0,125\cdot 2,0833251)-(1,118033989+0,25\cdot 0,750488294)=}
=
2
(
8
,
062257748
+
0
,
260415637
)
−
(
1
,
118033989
+
0
,
187622073
)
=
16
,
64534677
−
1
,
305656063
=
15
,
33969071.
{\displaystyle =2(8,062257748+0,260415637)-(1,118033989+0,187622073)=16,64534677-1,305656063=15,33969071.}
Palyginimui, tiesios linijos ilgis nuo taško (1; 1) iki taško (4; 16) yra
c
=
3
2
+
15
2
=
234
=
15
,
29705854.
{\displaystyle c={\sqrt {3^{2}+15^{2}}}={\sqrt {234}}=15,29705854.}
Apskaičiuosime parabolės
y
=
(
x
+
1
)
3
{\displaystyle y={\sqrt {(x+1)^{3}}}}
4
≤
x
≤
12.
{\displaystyle 4\leq x\leq 12.}
Randame
y
′
=
3
2
x
+
1
,
d
s
=
1
+
(
d
y
d
x
)
2
d
x
=
1
+
9
4
(
x
+
1
)
d
x
=
13
4
+
9
4
x
d
x
.
{\displaystyle y'={3 \over 2}{\sqrt {x+1}},\;ds={\sqrt {1+({dy \over dx})^{2}}}dx={\sqrt {1+{9 \over 4}(x+1)}}dx={\sqrt {{13 \over 4}+{9 \over 4}x}}dx.}
integralų lentelės
L
=
∫
L
d
s
=
∫
4
12
13
4
+
9
4
x
d
x
=
∫
4
12
1
4
⋅
13
+
9
x
d
x
=
1
9
⋅
1
2
∫
4
12
13
+
9
x
d
(
13
+
9
x
)
=
{\displaystyle L=\int _{L}ds=\int _{4}^{12}{\sqrt {{13 \over 4}+{9 \over 4}x}}\;dx=\int _{4}^{12}{\sqrt {1 \over 4}}\cdot {\sqrt {13+9x}}dx={1 \over 9}\cdot {1 \over 2}\int _{4}^{12}{\sqrt {13+9x}}d(13+9x)=}
=
1
18
⋅
(
13
+
9
x
)
1
2
+
1
3
2
|
4
12
=
{\displaystyle ={1 \over 18}\cdot {(13+9x)^{{1 \over 2}+1} \over {3 \over 2}}|_{4}^{12}=}
=
2
18
⋅
3
(
13
+
9
x
)
3
|
4
12
=
1
27
[
(
13
+
9
⋅
12
)
3
−
(
13
+
9
⋅
4
)
3
]
=
{\displaystyle ={2 \over 18\cdot 3}{\sqrt {(13+9x)^{3}}}|_{4}^{12}={1 \over 27}[{\sqrt {(13+9\cdot 12)^{3}}}-{\sqrt {(13+9\cdot 4)^{3}}}]=}
=
1
27
[
1771561
−
117649
]
=
1
27
⋅
(
1331
−
343
)
=
988
27
=≈
36
,
59259259.
{\displaystyle ={1 \over 27}[{\sqrt {1771561}}-{\sqrt {117649}}]={1 \over 27}\cdot (1331-343)={988 \over 27}=\approx 36,59259259.}
(
4
+
1
)
3
/
2
{\displaystyle (4+1)^{3/2}}
(
12
+
1
)
3
/
2
{\displaystyle (12+1)^{3/2}}
c
=
(
12
−
4
)
2
+
[
(
12
+
1
)
3
−
(
4
+
1
)
3
]
2
=
{\displaystyle c={\sqrt {(12-4)^{2}+[{\sqrt {(12+1)^{3}}}-{\sqrt {(4+1)^{3}}}]^{2}}}=}
=
8
2
+
(
2197
−
125
)
2
=
64
+
1273
,
906493
=
36
,
57740413.
{\displaystyle ={\sqrt {8^{2}+({\sqrt {2197}}-{\sqrt {125}})^{2}}}={\sqrt {64+1273,906493}}=36,57740413.}
Apskaičiuosime kreivės
y
=
x
{\displaystyle y={\sqrt {x}}}
1
≤
x
≤
16.
{\displaystyle 1\leq x\leq 16.}
Randame
y
′
=
(
x
1
2
)
′
=
1
2
⋅
1
x
.
{\displaystyle y'=(x^{1 \over 2})'={1 \over 2}\cdot {1 \over {\sqrt {x}}}.}
L
=
∫
1
16
1
+
(
y
′
)
2
d
x
=
∫
1
16
1
+
(
1
2
x
)
2
d
x
=
∫
1
16
1
+
1
4
x
d
x
=
1
2
∫
1
16
4
+
1
x
d
x
=
{\displaystyle L=\int _{1}^{16}{\sqrt {1+(y')^{2}}}dx=\int _{1}^{16}{\sqrt {1+({1 \over 2{\sqrt {x}}})^{2}}}dx=\int _{1}^{16}{\sqrt {1+{1 \over 4x}}}dx={1 \over 2}\int _{1}^{16}{\sqrt {4+{1 \over x}}}dx=}
=
1
2
[
x
1
x
+
4
+
1
4
ln
(
4
x
(
1
x
+
4
+
2
)
+
1
)
]
|
1
16
=
{\displaystyle ={1 \over 2}[x{\sqrt {{1 \over x}+4}}+{1 \over 4}\ln(4x({\sqrt {{1 \over x}+4}}+2)+1)]|_{1}^{16}=}
=
1
2
[
16
1
16
+
4
+
1
4
ln
(
4
⋅
16
(
1
16
+
4
+
2
)
+
1
)
]
−
1
2
[
1
1
1
+
4
+
1
4
ln
(
4
(
1
1
+
4
+
2
)
+
1
)
]
=
{\displaystyle ={1 \over 2}[16{\sqrt {{1 \over 16}+4}}+{1 \over 4}\ln(4\cdot 16({\sqrt {{1 \over 16}+4}}+2)+1)]-{1 \over 2}[1{\sqrt {{1 \over 1}+4}}+{1 \over 4}\ln(4({\sqrt {{1 \over 1}+4}}+2)+1)]=}
=
1
2
[
16
⋅
2
,
015564437
+
1
4
ln
(
64
(
2
,
015564437
+
2
)
+
1
)
]
−
1
2
[
5
+
1
4
ln
(
4
(
5
+
2
)
+
1
)
]
=
{\displaystyle ={1 \over 2}[16\cdot 2,015564437+{1 \over 4}\ln(64(2,015564437+2)+1)]-{1 \over 2}[{\sqrt {5}}+{1 \over 4}\ln(4({\sqrt {5}}+2)+1)]=}
=
1
2
[
32
,
24903099
+
1
4
ln
(
256
,
996124
+
1
)
]
−
1
2
[
2
,
236067978
+
1
4
ln
(
17
,
94427191
)
]
=
{\displaystyle ={1 \over 2}[32,24903099+{1 \over 4}\ln(256,996124+1)]-{1 \over 2}[2,236067978+{1 \over 4}\ln(17,94427191)]=}
=
1
2
[
32
,
24903099
+
1
4
⋅
5
,
552944561
]
−
1
2
[
2
,
236067978
+
2
,
88727095
4
]
=
{\displaystyle ={1 \over 2}[32,24903099+{1 \over 4}\cdot 5,552944561]-{1 \over 2}[2,236067978+{2,88727095 \over 4}]=}
=
16
,
81863357
−
1
,
478942858
=
15
,
33969071.
{\displaystyle =16,81863357-1,478942858=15,33969071.}
Palyginimui, tiesės ilgis nuo taško (1; 1) iki taško (16; 4) yra
c
=
(
16
−
1
)
2
+
(
4
−
1
)
2
=
225
+
9
=
15
,
29705854.
{\displaystyle c={\sqrt {(16-1)^{2}+(4-1)^{2}}}={\sqrt {225+9}}=15,29705854.}
Apskaičiuosime kreivės lanko ilgį , kai
y
=
1
−
ln
(
cos
x
)
;
{\displaystyle y=1-\ln(\cos x);}
0
≤
x
≤
π
3
.
{\displaystyle 0\leq x\leq {\pi \over 3}.}
y
′
=
(
1
−
ln
(
cos
x
)
)
′
=
−
−
sin
(
x
)
cos
(
x
)
=
sin
x
cos
x
.
{\displaystyle y'=(1-\ln(\cos x))'=-{\frac {-\sin(x)}{\cos(x)}}={\frac {\sin x}{\cos x}}.}
l
=
∫
0
π
3
1
+
sin
2
x
cos
2
x
d
x
=
∫
0
π
3
d
x
cos
x
=
ln
|
tan
(
x
2
+
π
4
)
|
|
0
π
3
=
ln
|
tan
5
π
12
|
−
ln
|
tan
π
4
|
=
1
,
316957897.
{\displaystyle l=\int _{0}^{\pi \over 3}{\sqrt {1+{\sin ^{2}x \over \cos ^{2}x}}}dx=\int _{0}^{\pi \over 3}{dx \over \cos x}=\ln \left|\tan \left({\frac {x}{2}}+{\frac {\pi }{4}}\right)\right||_{0}^{\pi \over 3}=\ln |\tan {5\pi \over 12}|-\ln |\tan {\pi \over 4}|=1,316957897.}
Apskaičiuokime cikloidės
x
=
a
(
t
−
sin
t
)
,
{\displaystyle x=a(t-\sin t),}
y
=
a
(
1
−
cos
t
)
{\displaystyle y=a(1-\cos t)}
(
a
>
0
)
{\displaystyle (a>0)}
Pirmoji cikloidės arka gaunama, kai parametras t kinta nuo 0 iki
2
π
.
{\displaystyle 2\pi .}
x
t
′
=
a
(
1
−
cos
t
)
,
{\displaystyle x_{t}'=a(1-\cos t),}
y
t
′
=
a
sin
t
,
{\displaystyle y_{t}'=a\sin t,}
x
t
′
2
+
y
t
′
2
=
a
2
(
1
−
2
cos
t
+
cos
2
t
)
+
a
2
sin
2
t
=
2
a
2
(
1
−
cos
t
)
=
{\displaystyle {\sqrt {x_{t}'^{2}+y_{t}'^{2}}}={\sqrt {a^{2}(1-2\cos t+\cos ^{2}t)+a^{2}\sin ^{2}t}}={\sqrt {2a^{2}(1-\cos t)}}=}
=
4
a
2
sin
2
t
t
2
=
2
a
|
sin
t
2
|
=
2
a
sin
t
2
,
{\displaystyle ={\sqrt {4a^{2}\sin ^{2}t{t \over 2}}}=2a|\sin {t \over 2}|=2a\sin {t \over 2},}
sin
t
2
≥
0
,
{\displaystyle \sin {t \over 2}\geq 0,}
t
∈
[
0
;
2
π
]
.
{\displaystyle t\in [0;2\pi ].}
L
=
2
a
∫
0
2
π
sin
t
2
d
t
=
4
a
∫
0
2
π
sin
t
2
d
(
t
/
2
)
=
−
4
a
cos
t
2
|
0
2
π
=
8
a
.
{\displaystyle L=2a\int _{0}^{2\pi }\sin {t \over 2}dt=4a\int _{0}^{2\pi }\sin {t \over 2}d(t/2)=-4a\cos {t \over 2}|_{0}^{2\pi }=8a.}
Kaip atrodo cikloidė galima pažiūrėti čia https://lt.wikipedia.org/wiki/Cikloidė
Rasime lanko AB ilgį susuktos linijos
x
=
cos
t
,
{\displaystyle x=\cos t,}
y
=
sin
t
,
{\displaystyle y=\sin t,}
z
=
2
t
,
{\displaystyle z=2t,}
0
≤
t
≤
π
.
{\displaystyle 0\leq t\leq \pi .}
s
=
∫
0
π
sin
2
t
+
cos
2
t
+
4
d
t
=
5
π
.
{\displaystyle s=\int _{0}^{\pi }{\sqrt {\sin ^{2}t+\cos ^{2}t+4}}dt={\sqrt {5}}\pi .}
Rasime lanko ilgį kardiodės
ρ
=
a
(
1
−
cos
ϕ
)
,
{\displaystyle \rho =a(1-\cos \phi ),}
a
>
0.
{\displaystyle a>0.}
s
=
∫
α
β
ρ
2
+
ρ
′
2
d
ϕ
=
2
a
∫
0
π
(
1
−
cos
ϕ
)
2
+
sin
2
ϕ
d
ϕ
=
{\displaystyle s=\int _{\alpha }^{\beta }{\sqrt {\rho ^{2}+\rho '^{2}}}d\phi =2a\int _{0}^{\pi }{\sqrt {(1-\cos \phi )^{2}+\sin ^{2}\phi }}d\phi =}
=
2
a
∫
0
π
1
−
2
cos
ϕ
+
cos
2
ϕ
+
sin
2
ϕ
d
ϕ
=
2
a
∫
0
π
2
(
1
−
cos
ϕ
)
d
ϕ
=
2
a
∫
0
π
4
sin
2
ϕ
2
d
ϕ
=
{\displaystyle =2a\int _{0}^{\pi }{\sqrt {1-2\cos \phi +\cos ^{2}\phi +\sin ^{2}\phi }}d\phi =2a\int _{0}^{\pi }{\sqrt {2(1-\cos \phi )}}d\phi =2a\int _{0}^{\pi }{\sqrt {4\sin ^{2}{\phi \over 2}}}d\phi =}
=
4
a
∫
0
π
sin
ϕ
2
=
−
8
a
cos
ϕ
2
|
0
π
=
−
8
a
(
0
−
1
)
=
8
a
.
{\displaystyle =4a\int _{0}^{\pi }\sin {\phi \over 2}=-8a\cos {\phi \over 2}|_{0}^{\pi }=-8a(0-1)=8a.}
Kaip atrodo kardioidė galima pažiūrėti čia https://en.wikipedia.org/wiki/Cardioid
Rasti kardiodės
ρ
=
a
(
1
+
cos
θ
)
{\displaystyle \rho =a(1+\cos \theta )}
Keisdami poliarinį kampą
θ
{\displaystyle \theta }
π
,
{\displaystyle \pi ,}
ρ
′
=
−
a
sin
θ
.
{\displaystyle \rho '=-a\sin \theta .}
s
=
2
∫
0
π
a
2
(
1
+
cos
θ
)
2
+
a
2
sin
2
θ
d
θ
=
2
a
∫
0
π
1
+
2
cos
θ
+
cos
2
θ
+
sin
2
θ
d
θ
=
{\displaystyle s=2\int _{0}^{\pi }{\sqrt {a^{2}(1+\cos \theta )^{2}+a^{2}\sin ^{2}\theta }}d\theta =2a\int _{0}^{\pi }{\sqrt {1+2\cos \theta +\cos ^{2}\theta +\sin ^{2}\theta }}d\theta =}
=
2
a
∫
0
π
2
+
2
cos
θ
d
θ
=
2
2
a
∫
0
π
1
+
cos
θ
d
θ
=
2
2
a
∫
0
π
2
cos
θ
2
d
θ
=
{\displaystyle =2a\int _{0}^{\pi }{\sqrt {2+2\cos \theta }}d\theta =2{\sqrt {2}}a\int _{0}^{\pi }{\sqrt {1+\cos \theta }}d\theta =2{\sqrt {2}}a\int _{0}^{\pi }{\sqrt {2}}\cos {\frac {\theta }{2}}\;d\theta =}
=
4
a
∫
0
π
cos
θ
2
d
θ
=
8
a
sin
θ
2
|
0
π
=
8
a
.
{\displaystyle =4a\int _{0}^{\pi }\cos {\frac {\theta }{2}}\;d\theta =8a\sin {\frac {\theta }{2}}|_{0}^{\pi }=8a.}
Rasime kreivės lanko ilgį, kai
ρ
=
sin
3
ϕ
3
,
0
≤
ϕ
≤
π
2
.
{\displaystyle \rho =\sin ^{3}{\phi \over 3},\;0\leq \phi \leq {\pi \over 2}.}
l
=
∫
0
π
3
(
sin
3
ϕ
3
)
2
+
(
3
sin
2
ϕ
3
⋅
1
3
cos
ϕ
3
)
2
d
ϕ
=
∫
0
π
3
sin
6
ϕ
3
+
sin
4
ϕ
3
⋅
cos
2
ϕ
3
d
ϕ
=
∫
0
π
2
sin
2
ϕ
3
sin
2
ϕ
3
+
cos
2
ϕ
3
d
ϕ
=
{\displaystyle l=\int _{0}^{\pi \over 3}{\sqrt {\left(\sin ^{3}{\phi \over 3}\right)^{2}+\left(3\sin ^{2}{\phi \over 3}\cdot {1 \over 3}\cos {\phi \over 3}\right)^{2}}}d\phi =\int _{0}^{\pi \over 3}{\sqrt {\sin ^{6}{\phi \over 3}+\sin ^{4}{\phi \over 3}\cdot \cos ^{2}{\phi \over 3}}}d\phi =\int _{0}^{\pi \over 2}\sin ^{2}{\phi \over 3}{\sqrt {\sin ^{2}{\phi \over 3}+\cos ^{2}{\phi \over 3}}}d\phi =}
=
1
2
∫
0
π
2
(
1
+
cos
2
ϕ
3
)
d
ϕ
=
1
2
⋅
π
2
+
1
2
⋅
3
2
sin
2
ϕ
3
|
0
π
2
=
π
4
+
3
4
⋅
3
2
=
π
4
+
3
3
8
.
{\displaystyle ={1 \over 2}\int _{0}^{\pi \over 2}(1+\cos {2\phi \over 3})d\phi ={1 \over 2}\cdot {\pi \over 2}+{1 \over 2}\cdot {3 \over 2}\sin {2\phi \over 3}|_{0}^{\pi \over 2}={\pi \over 4}+{3 \over 4}\cdot {{\sqrt {3}} \over 2}={\pi \over 4}+{3{\sqrt {3}} \over 8}.}
Archimedo spiralė. Apskaičiuosime ilgį pirmos vijos Archimedo spiralės:
ρ
=
a
ϕ
.
{\displaystyle \rho =a\phi .}
Pirma vija spiralės pasidaro, keičiantis poliariniui kampui
ϕ
{\displaystyle \phi }
2
π
.
{\displaystyle 2\pi .}
L
=
∫
α
β
ρ
(
ϕ
)
+
ρ
′
2
(
ϕ
)
d
ϕ
=
∫
0
2
π
a
2
ϕ
2
+
a
2
d
ϕ
=
a
∫
0
2
π
ϕ
2
+
1
d
ϕ
=
{\displaystyle L=\int _{\alpha }^{\beta }{\sqrt {\rho (\phi )+\rho '^{2}(\phi )}}d\phi =\int _{0}^{2\pi }{\sqrt {a^{2}\phi ^{2}+a^{2}}}d\phi =a\int _{0}^{2\pi }{\sqrt {\phi ^{2}+1}}d\phi =}
=
a
[
π
4
π
2
+
1
+
1
2
ln
(
2
π
+
4
π
2
+
1
)
]
=
{\displaystyle =a[\pi {\sqrt {4\pi ^{2}+1}}+{1 \over 2}\ln(2\pi +{\sqrt {4\pi ^{2}+1}})]=}
=
a
[
19
,
9876454
+
1
,
268648751
]
=
a
21
,
25629415.
{\displaystyle =a[19,9876454+1,268648751]=a21,25629415.}
Apskaičiuosime vienos vijos linijos ilgį:
x
=
cos
t
,
{\displaystyle x=\cos t,}
y
=
sin
t
,
{\displaystyle y=\sin t,}
z
=
t
,
{\displaystyle z=t,}
0
≤
t
≤
2
π
.
{\displaystyle 0\leq t\leq 2\pi .}
t . Gauname:
d
x
=
−
sin
(
t
)
d
t
,
{\displaystyle dx=-\sin(t)dt,}
d
y
=
cos
(
t
)
d
t
{\displaystyle dy=\cos(t)dt}
d
z
=
d
t
{\displaystyle dz=dt}
d
z
d
t
=
1
;
{\displaystyle {\frac {dz}{dt}}=1;}
(
x
t
′
)
2
+
(
y
t
′
)
2
+
(
z
t
′
)
2
=
(
−
sin
t
)
2
+
(
cos
t
)
2
+
1
.
{\displaystyle {\sqrt {(x_{t}')^{2}+(y_{t}')^{2}+(z_{t}')^{2}}}={\sqrt {(-\sin t)^{2}+(\cos t)^{2}+1}}.}
Randame vienos vijos ilgį:
L
=
∫
0
2
π
(
−
sin
t
)
2
+
(
cos
t
)
2
+
1
d
t
=
2
∫
0
2
π
d
t
=
2
t
|
0
2
π
=
2
2
π
.
{\displaystyle L=\int _{0}^{2\pi }{\sqrt {(-\sin t)^{2}+(\cos t)^{2}+1}}dt={\sqrt {2}}\int _{0}^{2\pi }dt={\sqrt {2}}t|_{0}^{2\pi }=2{\sqrt {2}}\pi .}
Apskaičiuoti ilgį hipocikloidės (astroidės):
x
=
a
cos
3
t
,
y
=
a
sin
3
t
.
{\displaystyle x=a\cos ^{3}t,\;\;y=a\sin ^{3}t.}
Sprendimas . Kadangi kreivė simetriška dviejų koordinačių ašių atžvilgiu, tai iš pradžių apskaičiuosime ketvirtadalį jos dalies, esančios pirmame ketvirtyje. Randame:
d
x
d
t
=
−
3
a
cos
2
t
sin
t
,
d
y
d
t
=
3
a
sin
2
t
cos
t
.
{\displaystyle {\frac {dx}{dt}}=-3a\cos ^{2}t\sin t,\;\;{\frac {dy}{dt}}=3a\sin ^{2}t\cos t.}
Parametras t kis nuo 0 iki
π
/
2.
{\displaystyle \pi /2.}
1
4
s
=
∫
0
π
/
2
9
a
2
cos
4
t
sin
2
t
+
9
a
2
sin
4
t
cos
2
t
d
t
=
3
a
∫
0
π
/
2
cos
2
t
sin
2
t
(
cos
2
t
+
sin
2
t
)
d
t
=
{\displaystyle {\frac {1}{4}}s=\int _{0}^{\pi /2}{\sqrt {9a^{2}\cos ^{4}t\sin ^{2}t+9a^{2}\sin ^{4}t\cos ^{2}t}}dt=3a\int _{0}^{\pi /2}{\sqrt {\cos ^{2}t\sin ^{2}t(\cos ^{2}t+\sin ^{2}t)}}dt=}
=
3
a
∫
0
π
/
2
cos
t
sin
t
d
t
=
3
a
∫
0
π
/
2
sin
t
d
(
sin
t
)
=
3
a
sin
2
t
2
|
0
π
/
2
=
3
a
2
;
s
=
6
a
.
{\displaystyle =3a\int _{0}^{\pi /2}\cos t\sin t\;dt=3a\int _{0}^{\pi /2}\sin t\;d(\sin t)=3a{\frac {\sin ^{2}t}{2}}|_{0}^{\pi /2}={\frac {3a}{2}};\;\;s=6a.}
Kaip atrodo astroidė galima pažiūrėti čia https://en.wikipedia.org/wiki/Astroid Kreivės masė nustatoma pagal formulę
m
=
∫
γ
1
+
(
y
′
)
2
d
x
,
{\displaystyle m=\int \gamma {\sqrt {1+(y')^{2}}}dx,}
čia
γ
{\displaystyle \gamma }
Nustatyti tiesės
y
=
3
−
3
x
5
{\displaystyle y=3-{\frac {3x}{5}}}
γ
{\displaystyle \gamma }
O ) didėja proporcingai, t. y.
γ
=
x
2
+
y
2
.
{\displaystyle \gamma ={\sqrt {x^{2}+y^{2}}}.}
Sprendimas . Pasinaudosime masės skaičiavimo formule
m
=
∫
L
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
x
2
+
y
2
1
+
[
y
′
]
2
d
x
=
{\displaystyle m=\int _{L}\gamma {\sqrt {1+[y']^{2}}}\;dx=\int _{0}^{5}{\sqrt {x^{2}+y^{2}}}{\sqrt {1+[y']^{2}}}\;dx=}
=
∫
0
5
x
2
+
(
3
−
3
x
5
)
2
1
+
(
−
3
5
)
2
d
x
=
{\displaystyle =\int _{0}^{5}{\sqrt {x^{2}+\left(3-{\frac {3x}{5}}\right)^{2}}}{\sqrt {1+\left(-{\frac {3}{5}}\right)^{2}}}\;dx=}
=
∫
0
5
x
2
+
9
−
18
x
5
+
9
x
2
25
1
+
9
25
d
x
=
{\displaystyle =\int _{0}^{5}{\sqrt {x^{2}+9-{\frac {18x}{5}}+{\frac {9x^{2}}{25}}}}{\sqrt {1+{\frac {9}{25}}}}\;dx=}
=
∫
0
5
9
−
18
x
5
+
34
x
2
25
34
25
d
x
=
{\displaystyle =\int _{0}^{5}{\sqrt {9-{\frac {18x}{5}}+{\frac {34x^{2}}{25}}}}{\sqrt {\frac {34}{25}}}\;dx=}
=
34
5
∫
0
5
34
25
x
2
−
18
5
x
+
9
d
x
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\int _{0}^{5}{\sqrt {{\frac {34}{25}}x^{2}-{\frac {18}{5}}x+9}}\;dx=}
=
34
5
∫
0
5
1.36
x
2
−
3.6
x
+
9
d
x
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\int _{0}^{5}{\sqrt {1.36x^{2}-3.6x+9}}\;dx=}
=
34
5
(
−
3.6
+
2
⋅
1.36
x
4
⋅
1.36
1.36
x
2
−
3.6
x
+
9
+
4
⋅
1.36
⋅
9
−
(
−
3.6
)
2
8
⋅
1.36
3
/
2
ln
|
2
⋅
1.36
x
−
3.6
+
2
1.36
(
1.36
x
2
−
3.6
x
+
9
)
|
)
|
0
5
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {-3.6+2\cdot 1.36x}{4\cdot 1.36}}{\sqrt {1.36x^{2}-3.6x+9}}+{\frac {4\cdot 1.36\cdot 9-(-3.6)^{2}}{8\cdot 1.36^{3/2}}}\ln |2\cdot 1.36x-3.6+2{\sqrt {1.36(1.36x^{2}-3.6x+9)}}|\right)|_{0}^{5}=}
=
34
5
(
−
3.6
+
2.72
x
5.44
1.36
x
2
−
3.6
x
+
9
+
48.96
−
12.96
8
⋅
2.515456
1
/
2
ln
|
2.72
x
−
3.6
+
2
1.36
(
1.36
x
2
−
3.6
x
+
9
)
|
)
|
0
5
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {-3.6+2.72x}{5.44}}{\sqrt {1.36x^{2}-3.6x+9}}+{\frac {48.96-12.96}{8\cdot 2.515456^{1/2}}}\ln |2.72x-3.6+2{\sqrt {1.36(1.36x^{2}-3.6x+9)}}|\right)|_{0}^{5}=}
=
34
5
(
−
3.6
+
2.72
x
5.44
1.36
x
2
−
3.6
x
+
9
+
36
12.68815132
ln
|
2.72
x
−
3.6
+
2
1.36
(
1.36
x
2
−
3.6
x
+
9
)
|
)
|
0
5
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {-3.6+2.72x}{5.44}}{\sqrt {1.36x^{2}-3.6x+9}}+{\frac {36}{12.68815132}}\ln |2.72x-3.6+2{\sqrt {1.36(1.36x^{2}-3.6x+9)}}|\right)|_{0}^{5}=}
=
34
5
(
−
3.6
+
2.72
⋅
5
5.44
1.36
⋅
25
−
3.6
⋅
5
+
9
+
2.8372927689
ln
|
2.72
⋅
5
−
3.6
+
2
1.36
(
1.36
⋅
25
−
3.6
⋅
5
+
9
)
|
)
−
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {-3.6+2.72\cdot 5}{5.44}}{\sqrt {1.36\cdot 25-3.6\cdot 5+9}}+2.8372927689\ln |2.72\cdot 5-3.6+2{\sqrt {1.36(1.36\cdot 25-3.6\cdot 5+9)}}|\right)-}
−
34
5
(
−
3.6
+
2.72
⋅
0
5.44
1.36
⋅
0
2
−
3.6
⋅
0
+
9
+
2.8372927689
ln
|
2.72
⋅
0
−
3.6
+
2
1.36
(
1.36
⋅
0
2
−
3.6
⋅
0
+
9
)
|
)
=
{\displaystyle -{\frac {\sqrt {34}}{5}}\left({\frac {-3.6+2.72\cdot 0}{5.44}}{\sqrt {1.36\cdot 0^{2}-3.6\cdot 0+9}}+2.8372927689\ln |2.72\cdot 0-3.6+2{\sqrt {1.36(1.36\cdot 0^{2}-3.6\cdot 0+9)}}|\right)=}
=
34
5
(
10
5.44
34
−
18
+
9
+
2.8372927689
ln
|
13.6
−
3.6
+
2
1.36
(
34
−
18
+
9
)
|
)
−
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {10}{5.44}}{\sqrt {34-18+9}}+2.8372927689\ln |13.6-3.6+2{\sqrt {1.36(34-18+9)}}|\right)-}
−
34
5
(
−
3.6
5.44
9
+
2.8372927689
ln
|
−
3.6
+
2
1.36
⋅
9
|
)
=
{\displaystyle -{\frac {\sqrt {34}}{5}}\left({\frac {-3.6}{5.44}}{\sqrt {9}}+2.8372927689\ln |-3.6+2{\sqrt {1.36\cdot 9}}|\right)=}
=
34
5
(
10
5.44
25
+
2.8372927689
ln
|
10
+
2
1.36
⋅
25
|
)
−
34
5
(
−
3.6
5.44
⋅
3
+
2.8372927689
ln
|
−
3.6
+
2
12.24
|
)
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {10}{5.44}}{\sqrt {25}}+2.8372927689\ln |10+2{\sqrt {1.36\cdot 25}}|\right)-{\frac {\sqrt {34}}{5}}\left({\frac {-3.6}{5.44}}\cdot 3+2.8372927689\ln |-3.6+2{\sqrt {12.24}}|\right)=}
=
34
5
(
50
5.44
+
2.8372927689
ln
|
10
+
2
34
|
)
−
34
5
(
−
10.8
5.44
+
2.8372927689
ln
|
−
3.6
+
2
12.24
|
)
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left({\frac {50}{5.44}}+2.8372927689\ln |10+2{\sqrt {34}}|\right)-{\frac {\sqrt {34}}{5}}\left({\frac {-10.8}{5.44}}+2.8372927689\ln |-3.6+2{\sqrt {12.24}}|\right)=}
=
34
5
(
9.191176471
+
2.8372927689
ln
|
21.66190379
|
)
−
34
5
(
−
10.8
5.44
+
2.8372927689
ln
|
−
3.6
+
2
12.24
|
)
=
{\displaystyle ={\frac {\sqrt {34}}{5}}\left(9.191176471+2.8372927689\ln |21.66190379|\right)-{\frac {\sqrt {34}}{5}}\left({\frac {-10.8}{5.44}}+2.8372927689\ln |-3.6+2{\sqrt {12.24}}|\right)=}
=
34
5
(
9.191176471
+
8.726250336
)
−
34
5
(
−
1.985294118
+
3.469823414
)
=
{\displaystyle ={\frac {\sqrt {34}}{5}}(9.191176471+8.726250336)-{\frac {\sqrt {34}}{5}}(-1.985294118+3.469823414)=}
=
34
5
⋅
17.91742681
−
34
5
⋅
1.484529296
=
16.43289751
34
5
=
19.16388698.
{\displaystyle ={\frac {\sqrt {34}}{5}}\cdot 17.91742681-{\frac {\sqrt {34}}{5}}\cdot 1.484529296={\frac {16.43289751{\sqrt {34}}}{5}}=19.16388698.}
Kad tą patį apskaičiuoti su programa "Free Pascal" reikia surasti tiesės ilgį, kai x kinta nuo 0 iki 5 (tai yra tiesės ilgis tik pirmame ketviryje):
l
=
5
2
+
3
2
=
25
+
9
=
34
=
5.830951895.
{\displaystyle l={\sqrt {5^{2}+3^{2}}}={\sqrt {25+9}}={\sqrt {34}}=5.830951895.}
Todėl "Free Pascal" kodas yra toks: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(a*0.000000003)+sqr((1000000001-a)*0.000000005));
writeln(sqrt(sqr(3)+sqr(5))*c/1000000000);
readln;
end.
duodantis rezultatą 19,163886990613093 po 18 sekundžių su 2,6 GHz procesoriumi.
Nustatyti parabolės
y
=
x
2
{\displaystyle y=x^{2}}
x kinta nuo 0 iki 5. Tiesės tankis
γ
{\displaystyle \gamma }
O ) didėja proporcingai, t. y.
γ
=
x
2
+
y
2
.
{\displaystyle \gamma ={\sqrt {x^{2}+y^{2}}}.}
Sprendimas .Pasirodo, integruojant taip ir taip gauname tokį patį rezultatą, kuris yra labai sudetingas ir ilgas. Net didžiausioje integralų lentelėje nėra kaip išintegruoti
∫
x
1
+
x
2
1
+
4
x
2
d
x
.
{\displaystyle \int x{\sqrt {1+x^{2}}}{\sqrt {1+4x^{2}}}dx.}
∫
a
+
b
x
c
+
p
x
d
x
,
{\displaystyle \int {\sqrt {a+bx}}{\sqrt {c+px}}dx,}
Free Pascal kodu. Free Pascal kodas, kuris skaičiuoja pagal formulę
m
=
∫
0
5
x
2
+
y
2
1
+
[
y
′
]
2
d
x
=
∫
0
5
x
2
+
(
x
2
)
2
1
+
(
2
x
)
2
d
x
,
{\displaystyle m=\int _{0}^{5}{\sqrt {x^{2}+y^{2}}}{\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}{\sqrt {x^{2}+(x^{2})^{2}}}{\sqrt {1+(2x)^{2}}}dx,}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+sqr(2*5.0*a/1000000000))*sqrt(sqr(5.0*a/1000000000)+sqr(sqr(5.0*a/1000000000)));
writeln(5*c/1000000000);
readln;
end
ir duoda atsakymą
m
=
327.860390075605
{\displaystyle m=327.860390075605}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+sqr(0.00000001*a))*sqrt(sqr(0.000000005*a)+sqr(sqr(0.000000005*a)));
writeln(0.000000005*c);
readln;
end.
duoda atsakymą
m
=
327.86039007560539
{\displaystyle m=327.86039007560539}
Kitoks kreivės masės apskaičiavimo Free Pascal kodas yra: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000005*a-0.000000005*(a-1))+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*sqrt(sqr(0.000000005*a)+sqr(sqr(0.000000005*a)));
writeln(c);
readln;
end.
kuris duoda atsakymą
m
=
327.860389859764
{\displaystyle m=327.860389859764}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000005)+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*sqrt(sqr(0.000000005*a)+sqr(sqr(0.000000005*a)));
writeln(c);
readln;
end
kuris duoda atsakymą
m
=
327.860389859763
{\displaystyle m=327.860389859763}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(0.000000000000000025+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*sqrt(sqr(0.000000005*a)+sqr(sqr(0.000000005*a)));
writeln(c);
readln;
end.
kuris duoda atsakymą
m
=
327.860389859763
{\displaystyle m=327.860389859763}
Free Pascal automatiškai optimizuoja kodą pakeldamas konstantą 0,000000005 kvadratu ir visoms iteracijoms naudodamas gautą 0,000000000000000025 reikšmę).
Nustatyti parabolės
y
=
x
2
{\displaystyle y=x^{2}}
x kinta nuo 0 iki 10. Tiesės tankis
γ
{\displaystyle \gamma }
O ) didėja proporcingai tik Ox kryptimi, t. y.
γ
=
x
.
{\displaystyle \gamma =x.}
Sprendimas . Greičiausias būdas apskaičiuoti, tai ko reikalauja sąlyga (uždavinys) yra toks:
m
=
∫
0
10
x
d
x
=
x
2
2
|
0
10
=
10
2
2
−
0
2
2
=
50.
{\displaystyle m=\int _{0}^{10}x\;dx={\frac {x^{2}}{2}}|_{0}^{10}={\frac {10^{2}}{2}}-{\frac {0^{2}}{2}}=50.}
Kitas būdas yra toks:
m
=
∫
0
10
γ
1
+
[
y
′
]
2
d
x
=
∫
0
10
x
1
+
(
2
x
)
2
d
x
=
∫
0
10
x
1
+
4
x
2
d
x
=
{\displaystyle m=\int _{0}^{10}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{10}x{\sqrt {1+(2x)^{2}}}dx=\int _{0}^{10}x{\sqrt {1+4x^{2}}}dx=}
=
∫
0
10
2
x
1
4
+
x
2
d
x
=
2
3
(
x
2
+
1
4
)
3
/
2
|
0
10
=
{\displaystyle =\int _{0}^{10}2x{\sqrt {{\frac {1}{4}}+x^{2}}}dx={\frac {2}{3}}(x^{2}+{\frac {1}{4}})^{3/2}|_{0}^{10}=}
=
2
3
(
10
2
+
1
4
)
3
/
2
−
2
3
(
0
2
+
1
4
)
3
/
2
=
{\displaystyle ={\frac {2}{3}}(10^{2}+{\frac {1}{4}})^{3/2}-{\frac {2}{3}}(0^{2}+{\frac {1}{4}})^{3/2}=}
=
2
3
⋅
100.25
3
/
2
−
2
3
(
1
2
)
3
=
{\displaystyle ={\frac {2}{3}}\cdot 100.25^{3/2}-{\frac {2}{3}}\left({\frac {1}{2}}\right)^{3}=}
=669,16822851623458973388183928978 - (2/3)*(1/8)=
=669,16822851623458973388183928978 - 1/12=
=669,08489518290125640054850595645;
čia pasinaudojome integralų lentele
∫
x
x
2
±
a
2
d
x
=
1
3
(
x
2
±
a
2
)
3
/
2
.
{\displaystyle \int x{\sqrt {x^{2}\pm a^{2}}}dx={\frac {1}{3}}(x^{2}\pm a^{2})^{3/2}.}
Tuo atveju, jeigu x kinta nuo 0 iki 5 tada:
m
=
∫
0
5
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
x
1
+
4
x
2
d
x
=
2
∫
0
5
x
1
4
+
x
2
d
x
=
{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}x{\sqrt {1+4x^{2}}}dx=2\int _{0}^{5}x{\sqrt {{\frac {1}{4}}+x^{2}}}dx=}
=
2
3
(
5
2
+
1
4
)
3
/
2
−
2
3
(
0
2
+
1
4
)
3
/
2
=
{\displaystyle ={\frac {2}{3}}(5^{2}+{\frac {1}{4}})^{3/2}-{\frac {2}{3}}(0^{2}+{\frac {1}{4}})^{3/2}=}
=
2
3
⋅
25.25
3
/
2
−
2
3
(
1
2
)
3
=
{\displaystyle ={\frac {2}{3}}\cdot 25.25^{3/2}-{\frac {2}{3}}\left({\frac {1}{2}}\right)^{3}=}
=84,586453144434159774345479682393-1/12=
=84,50311981110082644101214634906.
Free Pascal kodas duodą tokį patį rezultatą (kai x kinta nuo 0 iki 5):var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000005)+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*0.000000005*a;
writeln(c);
readln;
end.
m=84,5031198757743 po 25 sekundžių su 2,6 GHz procesoriumi.
Alternatyvus Free Pascal kodas, skaičiuojantis pagal formulę
m
=
∫
0
5
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
x
1
+
4
x
2
d
x
{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}x{\sqrt {1+4x^{2}}}dx}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+4*sqr(5.0*a/1000000000))*a*5/1000000000;
writeln(c*5/1000000000);
readln;
end.
duodantis atsakymą m=84,5031199367086 po 25 sekundžių su 2,6 GHz procesoriumi. Optimizuotas jo variantas: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+4*sqr(5.0*a/1000000000))*a;
writeln(c*sqr(5/1000000000));
readln;
end.
duoda atsakymą m=84,503119936731021 po 23 sekundžių su 2,6 GHz procesoriumi. Dar labiau optimizuotas jo variantas: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+4*sqr(0.000000005*a))*a;
writeln(c*sqr(5/1000000000));
readln;
end.
duoda atsakymą m=84,503119936731021 po 17 sekundžių su 2,6 GHz procesoriumi (vadinasi, 1000000000 dalybos operacijų padaroma per 23-17=6 sekundes su 2,6 GHz procesoriumi; tačiau panaudojus šį kodą: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+1/a;
writeln(c);
readln;
end.
gauname atsakymą 21,3004815025070 po 8 sekundžių su 2,6 GHz procesoriumi (beje,
∫
1
10
9
1
x
d
x
=
ln
(
10
9
)
−
ln
(
1
)
=
9
ln
(
10
)
−
0
=
20.7232658369464
{\displaystyle \int _{1}^{10^{9}}{\frac {1}{x}}\;dx=\ln(10^{9})-\ln(1)=9\ln(10)-0=20.7232658369464}
Nustatyti parabolės
y
=
x
2
{\displaystyle y=x^{2}}
x kinta nuo 0 iki 5. Tiesės tankis
γ
{\displaystyle \gamma }
O ) didėja proporcingai Ox kryptimi ir Oy kryptimi, t. y.
γ
=
x
+
y
.
{\displaystyle \gamma =x+y.}
Sprendimas .
y
′
=
(
x
2
)
′
=
2
x
;
{\displaystyle y'=(x^{2})'=2x;}
m
=
∫
0
5
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
(
x
+
y
)
1
+
[
y
′
]
2
d
x
=
∫
0
5
(
x
+
x
2
)
1
+
4
x
2
d
x
=
2
∫
0
5
x
(
1
+
x
)
1
4
+
x
2
d
x
;
{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+y){\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2}){\sqrt {1+4x^{2}}}dx=2\int _{0}^{5}x(1+x){\sqrt {{\frac {1}{4}}+x^{2}}}dx;}
Toliau pasinaudodami Wolframo internetiniu integratoriumi gauname , kad :
m
=
∫
0
5
(
x
+
x
2
)
1
+
4
x
2
d
x
=
(
1
96
4
x
2
+
1
(
24
x
3
+
32
x
2
+
3
x
+
8
)
−
1
64
arcsinh
(
2
x
)
)
|
0
5
=
{\displaystyle m=\int _{0}^{5}(x+x^{2}){\sqrt {1+4x^{2}}}dx=\left({\frac {1}{96}}{\sqrt {4x^{2}+1}}(24x^{3}+32x^{2}+3x+8)-{\frac {1}{64}}{\text{arcsinh}}(2x)\right)|_{0}^{5}=}
=
(
1
96
4
⋅
5
2
+
1
(
24
⋅
5
3
+
32
⋅
5
2
+
3
⋅
5
+
8
)
−
1
64
arcsinh
(
2
⋅
5
)
)
−
(
1
96
4
⋅
0
2
+
1
(
24
⋅
0
3
+
32
⋅
0
2
+
3
⋅
0
+
8
)
−
1
64
arcsinh
(
2
⋅
0
)
)
=
{\displaystyle =\left({\frac {1}{96}}{\sqrt {4\cdot 5^{2}+1}}(24\cdot 5^{3}+32\cdot 5^{2}+3\cdot 5+8)-{\frac {1}{64}}{\text{arcsinh}}(2\cdot 5)\right)-\left({\frac {1}{96}}{\sqrt {4\cdot 0^{2}+1}}(24\cdot 0^{3}+32\cdot 0^{2}+3\cdot 0+8)-{\frac {1}{64}}{\text{arcsinh}}(2\cdot 0)\right)=}
=
(
1
96
101
(
24
⋅
125
+
32
⋅
25
+
15
+
8
)
−
1
64
arcsinh
(
10
)
)
−
(
1
96
1
⋅
8
−
1
64
arcsinh
(
0
)
)
=
{\displaystyle =\left({\frac {1}{96}}{\sqrt {101}}(24\cdot 125+32\cdot 25+15+8)-{\frac {1}{64}}{\text{arcsinh}}(10)\right)-\left({\frac {1}{96}}{\sqrt {1}}\cdot 8-{\frac {1}{64}}{\text{arcsinh}}(0)\right)=}
=
(
1
96
101
(
3000
+
800
+
15
+
8
)
−
1
64
arcsinh
(
10
)
)
−
(
1
12
−
1
64
arcsinh
(
0
)
)
=
{\displaystyle =\left({\frac {1}{96}}{\sqrt {101}}(3000+800+15+8)-{\frac {1}{64}}{\text{arcsinh}}(10)\right)-\left({\frac {1}{12}}-{\frac {1}{64}}{\text{arcsinh}}(0)\right)=}
=
(
3823
96
101
−
1
64
arcsinh
(
10
)
)
−
(
1
12
−
1
64
arcsinh
(
0
)
)
=
{\displaystyle =\left({\frac {3823}{96}}{\sqrt {101}}-{\frac {1}{64}}{\text{arcsinh}}(10)\right)-\left({\frac {1}{12}}-{\frac {1}{64}}{\text{arcsinh}}(0)\right)=}
=(400,21535937026211982341926834875-0,04684723359840577716947805527494)-(0,08333333333333333333333333333333-0)=
=400,16851213666371404624979029348-0,08333333333333333333333333333333=400,08517880333038071291645696014.
Free Pascal kodas: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000005)+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*(0.000000005*a+sqr(0.000000005*a));
writeln(c);
readln;
end.
duoda atsakymą m=400,085179290551 po 27 sekundžių su 2,6 GHz procesoriumi.
Nustatyti parabolės
y
=
x
2
{\displaystyle y=x^{2}}
x kinta nuo 0 iki 5. Tiesės tankis
γ
{\displaystyle \gamma }
O ) didėja pagal formulę
γ
=
(
x
+
y
)
2
.
{\displaystyle \gamma =(x+y)^{2}.}
Sprendimas . Pasinaudodami internetiniu integratoriumi , gauname:
m
=
∫
0
5
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
(
x
+
y
)
2
1
+
[
y
′
]
2
d
x
=
∫
0
5
(
x
+
x
2
)
2
1
+
4
x
2
d
x
=
{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+y)^{2}{\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2})^{2}{\sqrt {1+4x^{2}}}dx=}
=
1
7680
(
2
4
x
2
+
1
(
640
x
5
+
1536
x
4
+
1000
x
3
+
128
x
2
+
105
x
−
64
)
−
105
arcsinh
(
2
x
)
)
|
0
5
=
{\displaystyle ={\frac {1}{7680}}\left(2{\sqrt {4x^{2}+1}}(640x^{5}+1536x^{4}+1000x^{3}+128x^{2}+105x-64)-105{\text{arcsinh}}(2x)\right)|_{0}^{5}=}
=
1
7680
(
2
101
(
640
⋅
3125
+
1536
⋅
625
+
1000
⋅
125
+
128
⋅
25
+
105
⋅
5
−
64
)
−
105
arcsinh
(
10
)
)
−
1
7680
(
2
1
⋅
(
−
64
)
−
105
arcsinh
(
0
)
)
=
{\displaystyle ={\frac {1}{7680}}\left(2{\sqrt {101}}(640\cdot 3125+1536\cdot 625+1000\cdot 125+128\cdot 25+105\cdot 5-64)-105{\text{arcsinh}}(10)\right)-{\frac {1}{7680}}\left(2{\sqrt {1}}\cdot (-64)-105{\text{arcsinh}}(0)\right)=}
=
1
7680
(
2
101
(
2000000
+
960000
+
125000
+
3200
+
525
−
64
)
−
105
arcsinh
(
10
)
)
−
1
7680
(
−
128
−
0
)
=
{\displaystyle ={\frac {1}{7680}}\left(2{\sqrt {101}}(2000000+960000+125000+3200+525-64)-105{\text{arcsinh}}(10)\right)-{\frac {1}{7680}}\left(-128-0\right)=}
=
1
7680
(
2
101
⋅
3088661
−
105
arcsinh
(
10
)
)
+
128
7680
=
{\displaystyle ={\frac {1}{7680}}\left(2{\sqrt {101}}\cdot 3088661-105{\text{arcsinh}}(10)\right)+{\frac {128}{7680}}=}
=
1
7680
(
62081317
,
771613740125811409969418
−
314
,
81340978128682257889253144763
)
+
128
7680
=
{\displaystyle ={\frac {1}{7680}}\left(62081317,771613740125811409969418-314,81340978128682257889253144763\right)+{\frac {128}{7680}}=}
=(62081002,958203958838988831076887+128)/7680=8083,4805935161404738266707131363.
Panaudojus Free Pascal kodą: var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000005*a-0.000000005*(a-1))+sqr(sqr(0.000000005*a)-sqr(0.000000005*(a-1))))*sqr(0.000000005*a+sqr(0.000000005*a));
writeln(c);
readln;
end.
gauname atsakymą m=8083,48061127561 po 30 sekundžių su 2,6 GHz procesoriumi.
Alternatyvus Free Pascal kodas, skaičiuojantis pagal formulę
m
=
∫
0
5
γ
1
+
[
y
′
]
2
d
x
=
∫
0
5
(
x
+
x
2
)
2
1
+
4
x
2
d
x
{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2})^{2}{\sqrt {1+4x^{2}}}dx}
var
a:longint;
c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(1+sqr(2*0.000000005*a))*sqr(0.000000005*a+sqr(0.000000005*a));
writeln(c*0.000000005);
readln;
end.
ir duoda atsakymą m=8083,4806161241980 po 22 sekundžių su 2,6 GHz procesoriumi. [ keisti ] Plotas sukant kokia nors funkcija (pavyzdžiui, parabolę ) aplink Ox ašį apskaičiuojamas pagal formule:
S
=
2
π
∫
a
b
f
(
x
)
1
+
(
f
′
(
x
)
)
2
d
x
.
{\displaystyle S=2\pi \int _{a}^{b}f(x){\sqrt {1+(f'(x))^{2}}}dx.}
Jeigu paviršius gaunamas sukimu aplink ašį Ox kreive AB , nusakomos parametrinėmis lygtimis
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
y
=
y
(
t
)
,
{\displaystyle y=y(t),}
α
≤
t
≤
β
,
{\displaystyle \alpha \leq t\leq \beta ,}
y
(
t
)
≥
0
,
{\displaystyle y(t)\geq 0,}
a iki b , keičiantis t nuo
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
x
(
α
)
=
a
,
{\displaystyle x(\alpha )=a,}
x
(
β
)
=
b
,
{\displaystyle x(\beta )=b,}
x
=
x
(
t
)
,
{\displaystyle x=x(t),}
S
=
2
π
∫
α
β
y
(
t
)
1
+
(
d
y
d
x
)
2
d
x
=
2
π
∫
α
β
y
(
t
)
x
′
2
(
t
)
+
y
′
2
(
t
)
d
t
.
{\displaystyle S=2\pi \int _{\alpha }^{\beta }y(t){\sqrt {1+({dy \over dx})^{2}}}dx=2\pi \int _{\alpha }^{\beta }y(t){\sqrt {x'^{2}(t)+y'^{2}(t)}}dt.}
Pagaliau, jeigu kreivė užduota lygtimi poliarinėse koordinatėse:
ρ
=
ρ
(
ϕ
)
,
{\displaystyle \rho =\rho (\phi ),}
α
≤
ϕ
≤
β
,
{\displaystyle \alpha \leq \phi \leq \beta ,}
ρ
(
ϕ
)
{\displaystyle \rho (\phi )}
[
α
,
β
]
,
{\displaystyle [\alpha ,\beta ],}
x
=
ρ
(
ϕ
)
cos
ϕ
,
{\displaystyle x=\rho (\phi )\cos \phi ,}
y
=
ρ
(
ϕ
)
sin
ϕ
,
{\displaystyle y=\rho (\phi )\sin \phi ,}
α
≤
ϕ
≤
β
,
{\displaystyle \alpha \leq \phi \leq \beta ,}
S
=
2
π
∫
α
β
ρ
(
ϕ
)
sin
ϕ
ρ
2
(
ϕ
)
+
ρ
′
2
(
ϕ
)
d
ϕ
.
{\displaystyle S=2\pi \int _{\alpha }^{\beta }\rho (\phi )\sin \phi {\sqrt {\rho ^{2}(\phi )+\rho '^{2}(\phi )}}d\phi .}
Sukimo paviršiaus ploto skaičiavimo esmė yra
C
=
2
π
⋅
R
{\displaystyle C=2\pi \cdot R}
R apskirtimo spindulys (R=f(x)). Tai tiesiog, tarsi, vidutinis kreivės [trumpas] atkarpos ilgis
(
l
k
+
1
−
l
k
=
Δ
l
)
{\displaystyle (l_{k+1}-l_{k}=\Delta l)}
R =y ir
2
π
{\displaystyle 2\pi }
C
=
2
π
R
=
2
π
y
{\displaystyle C=2\pi R=2\pi y}
Δ
l
{\displaystyle \Delta l}
h
=
Δ
l
{\displaystyle h=\Delta l}
Δ
l
⋅
2
π
y
=
h
⋅
2
π
f
(
x
)
,
{\displaystyle \Delta l\cdot 2\pi y=h\cdot 2\pi f(x),}
Ox ašį. Toliau gaunama nauja funkcija
2
π
⋅
f
(
x
)
1
+
(
f
′
(
x
)
)
2
,
{\displaystyle 2\pi \cdot f(x){\sqrt {1+(f'(x))^{2}}},}
a iki b . Čia
∫
a
b
1
+
(
f
′
(
x
)
)
2
d
x
{\displaystyle \int _{a}^{b}{\sqrt {1+(f'(x))^{2}}}dx}
x kinta nuo a iki b . Apskaičiuosime plotą S paviršiaus rutulinio pusiaujo, atsiradusio dėl sukimo pusiauapskritimio,
f
(
x
)
=
R
2
−
x
2
,
−
R
<
a
≤
x
≤
b
<
R
,
{\displaystyle f(x)={\sqrt {R^{2}-x^{2}}},\;-R<a\leq x\leq b<R,}
Ox . Pagal pirmą formulę gauname
S
=
2
π
∫
a
b
R
2
−
x
2
1
+
x
2
R
2
−
x
2
d
x
=
2
π
∫
a
b
R
2
−
x
2
R
2
R
2
−
x
2
d
x
=
2
π
∫
a
b
R
d
x
=
2
π
R
x
|
a
b
=
2
π
R
(
b
−
a
)
=
2
π
R
h
.
{\displaystyle S=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {1+{x^{2} \over R^{2}-x^{2}}}}dx=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {R^{2} \over R^{2}-x^{2}}}dx=2\pi \int _{a}^{b}Rdx=2\pi Rx|_{a}^{b}=2\pi R(b-a)=2\pi Rh.}
Apskaičiuosime 1/2 rutulio paviršiaus ploto, atsiradusio dėl sukimo pusiauapskritimio (sukama 1/4 apskritimo aplink Ox ašį),
f
(
x
)
=
R
2
−
x
2
,
0
≤
x
≤
R
,
{\displaystyle f(x)={\sqrt {R^{2}-x^{2}}},\;0\leq x\leq R,}
Ox , kai R=3. Pagal pirmą formulę gauname
S
=
2
π
∫
a
b
R
2
−
x
2
1
+
x
2
R
2
−
x
2
d
x
=
2
π
∫
0
R
R
2
−
x
2
R
2
R
2
−
x
2
d
x
=
2
π
∫
0
R
R
d
x
=
2
π
R
⋅
x
|
0
R
=
2
π
R
(
R
−
0
)
=
2
π
R
2
=
2
π
⋅
3
2
=
18
π
=
56.54866776.
{\displaystyle S=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {1+{x^{2} \over R^{2}-x^{2}}}}dx=2\pi \int _{0}^{R}{\sqrt {R^{2}-x^{2}}}{\sqrt {R^{2} \over R^{2}-x^{2}}}dx=2\pi \int _{0}^{R}Rdx=2\pi R\cdot x|_{0}^{R}=2\pi R(R-0)=2\pi R^{2}=2\pi \cdot 3^{2}=18\pi =56.54866776.}
Išvada yra paprasta ir akį režianti: kokį atstuma neimsi kiekviename intervale, ar tai būtų R-2=3-2=1 ar tai būtų 2-1=1, kai R=3, bet paviršiaus plotas visada bus toks pat ir kas svarbiausia teisingas. Nes kai tolstama nuo centro (kai 2 taškai kurie sudaro kreivę link R artėja) tai nuožulnesnis paviršius gaunamas (ilgesnė kreivė-linija, kuri bus sukama aplink Ox ašį), bet trumpesnio ilgio apskritimas
c
=
2
π
R
{\displaystyle c=2\pi R}
Ox ašį. Ir vienas kitą kompensuoja ir visada gaunasi vienodai, nepriklausomai nuo to kokioje srityje paimsi reikšmes (b-a), kurios yra 2 taškai ant Ox ašies. Apskaičiuosime rutulio paviršiaus plotą, atsiradusio dėl sukimo pusiauapskritimio,
f
(
x
)
=
R
2
−
x
2
,
1
≤
x
≤
3
,
{\displaystyle f(x)={\sqrt {R^{2}-x^{2}}},\;1\leq x\leq 3,}
Ox , kai R=3. Pagal pirmą formulę gauname
S
=
2
π
∫
a
b
R
2
−
x
2
1
+
x
2
R
2
−
x
2
d
x
=
2
π
∫
1
3
R
2
−
x
2
R
2
R
2
−
x
2
d
x
=
2
π
∫
1
3
R
d
x
=
2
π
R
(
3
−
1
)
=
4
π
R
=
2
π
⋅
3
⋅
2
=
12
π
=
37.69911184.
{\displaystyle S=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {1+{x^{2} \over R^{2}-x^{2}}}}dx=2\pi \int _{1}^{3}{\sqrt {R^{2}-x^{2}}}{\sqrt {R^{2} \over R^{2}-x^{2}}}dx=2\pi \int _{1}^{3}Rdx=2\pi R(3-1)=4\pi R=2\pi \cdot 3\cdot 2=12\pi =37.69911184.}
Patikriname, kad jei visas rutlio paviršiaus plotas yra
4
π
R
2
=
4
π
⋅
3
2
=
36
π
{\displaystyle 4\pi R^{2}=4\pi \cdot 3^{2}=36\pi }
1
4
{\displaystyle {\frac {1}{4}}}
1
4
⋅
4
π
3
2
=
π
9.
{\displaystyle {\frac {1}{4}}\cdot 4\pi 3^{2}=\pi 9.}
x negali būti daugiau už vieną ir mažiau už -1. Apskaičiuosime rutulio paviršiaus plotą, atsiradusio dėl sukimo pusiauapskritimio,
f
(
x
)
=
R
2
−
x
2
,
0
≤
x
≤
2
,
{\displaystyle f(x)={\sqrt {R^{2}-x^{2}}},\;0\leq x\leq 2,}
Ox , kai R=3. Pagal pirmą formulę gauname
S
=
2
π
∫
a
b
R
2
−
x
2
1
+
x
2
R
2
−
x
2
d
x
=
2
π
∫
0
1
R
2
−
x
2
R
2
R
2
−
x
2
d
x
=
2
π
∫
0
2
R
d
x
=
2
π
R
(
2
−
0
)
=
4
π
3
=
12
π
=
37.69911184.
{\displaystyle S=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {1+{x^{2} \over R^{2}-x^{2}}}}dx=2\pi \int _{0}^{1}{\sqrt {R^{2}-x^{2}}}{\sqrt {R^{2} \over R^{2}-x^{2}}}dx=2\pi \int _{0}^{2}Rdx=2\pi R(2-0)=4\pi 3=12\pi =37.69911184.}
Apskaičiuosime visą rutulio paviršiaus plotą, atsiradusio dėl sukimo pusiauapskritimio,
f
(
x
)
=
R
2
−
x
2
,
−
3
≤
x
≤
3
,
{\displaystyle f(x)={\sqrt {R^{2}-x^{2}}},\;-3\leq x\leq 3,}
Ox , kai R=3. Pagal pirmą formulę gauname
S
=
2
π
∫
a
b
R
2
−
x
2
1
+
x
2
R
2
−
x
2
d
x
=
2
π
∫
−
3
3
R
2
−
x
2
R
2
R
2
−
x
2
d
x
=
2
π
∫
−
3
3
R
d
x
=
2
π
R
x
|
−
3
3
=
2
π
R
(
3
−
(
−
3
)
)
=
2
π
⋅
3
⋅
6
=
36
π
=
113.0973355.
{\displaystyle S=2\pi \int _{a}^{b}{\sqrt {R^{2}-x^{2}}}{\sqrt {1+{x^{2} \over R^{2}-x^{2}}}}dx=2\pi \int _{-3}^{3}{\sqrt {R^{2}-x^{2}}}{\sqrt {R^{2} \over R^{2}-x^{2}}}dx=2\pi \int _{-3}^{3}Rdx=2\pi Rx|_{-3}^{3}=2\pi R(3-(-3))=2\pi \cdot 3\cdot 6=36\pi =113.0973355.}
Apskaičiuoti paraboloido paviršiaus plotą, atsiradusio dėl sukimo aplink Ox ašį parabolės
y
2
=
2
p
x
{\displaystyle y^{2}=2px}
x kitimosi nuo
x
=
0
{\displaystyle x=0}
x
=
k
{\displaystyle x=k}
y
=
2
p
x
,
y
′
=
2
p
2
x
,
1
+
(
y
′
)
2
=
1
+
2
p
4
x
=
2
x
+
p
2
x
.
{\displaystyle y={\sqrt {2px}},\quad y'={\frac {\sqrt {2p}}{2{\sqrt {x}}}},\quad {\sqrt {1+(y')^{2}}}={\sqrt {1+{\frac {2p}{4x}}}}={\sqrt {\frac {2x+p}{2x}}}.}
S
=
2
π
∫
a
b
f
(
x
)
1
+
(
f
′
(
x
)
)
2
d
x
=
2
π
∫
0
k
2
p
x
1
+
(
2
p
2
x
)
2
d
x
=
2
π
∫
0
k
2
p
x
1
+
2
p
4
x
d
x
=
2
π
∫
0
k
2
p
x
2
x
+
p
2
x
d
x
=
{\displaystyle S=2\pi \int _{a}^{b}f(x){\sqrt {1+(f'(x))^{2}}}dx=2\pi \int _{0}^{k}{\sqrt {2px}}{\sqrt {1+({\frac {\sqrt {2p}}{2{\sqrt {x}}}})^{2}}}dx=2\pi \int _{0}^{k}{\sqrt {2px}}{\sqrt {1+{\frac {2p}{4x}}}}dx=2\pi \int _{0}^{k}{\sqrt {2px}}{\sqrt {\frac {2x+p}{2x}}}dx=}
=
2
π
p
∫
0
k
2
x
+
p
d
x
=
π
p
∫
0
k
2
x
+
p
d
(
2
x
+
p
)
=
π
p
⋅
(
2
x
+
p
)
3
3
/
2
|
0
k
=
2
π
p
3
⋅
[
(
2
k
+
p
)
3
2
−
p
3
2
]
,
{\displaystyle =2\pi {\sqrt {p}}\int _{0}^{k}{\sqrt {2x+p}}dx=\pi {\sqrt {p}}\int _{0}^{k}{\sqrt {2x+p}}d(2x+p)=\pi {\sqrt {p}}\cdot {\frac {\sqrt {(2x+p)^{3}}}{3/2}}|_{0}^{k}={\frac {2\pi {\sqrt {p}}}{3}}\cdot [(2k+p)^{3 \over 2}-p^{3 \over 2}],}
kur d(2x+p)=2dx; dx=(d(2x+p))/2.
Apskaičiuoti paraboloido paviršiaus plotą, atsiradusio dėl sukimo aplink Ox ašį parabolės
y
2
=
x
{\displaystyle y^{2}=x}
x kitimosi nuo
x
=
0
{\displaystyle x=0}
x
=
4
{\displaystyle x=4}
y
=
x
,
y
′
=
1
2
x
.
{\displaystyle y={\sqrt {x}},\quad y'={\frac {1}{2{\sqrt {x}}}}.}
S
=
2
π
∫
a
b
f
(
x
)
1
+
(
f
′
(
x
)
)
2
d
x
=
2
π
∫
0
4
x
1
+
(
1
2
x
)
2
d
x
=
2
π
∫
0
4
x
1
+
1
4
x
d
x
=
2
π
∫
0
4
x
4
x
+
1
4
x
d
x
=
{\displaystyle S=2\pi \int _{a}^{b}f(x){\sqrt {1+(f'(x))^{2}}}dx=2\pi \int _{0}^{4}{\sqrt {x}}{\sqrt {1+({\frac {1}{2{\sqrt {x}}}})^{2}}}dx=2\pi \int _{0}^{4}{\sqrt {x}}{\sqrt {1+{\frac {1}{4x}}}}dx=2\pi \int _{0}^{4}{\sqrt {x}}{\sqrt {\frac {4x+1}{4x}}}dx=}
=
2
π
∫
0
4
4
x
+
1
4
d
x
=
2
π
∫
0
4
1
2
⋅
4
x
+
1
d
x
=
π
∫
0
4
4
x
+
1
d
x
=
π
∫
0
4
4
x
+
1
d
(
2
x
+
p
)
2
=
π
2
⋅
(
4
x
+
1
)
3
3
2
|
0
4
=
{\displaystyle =2\pi \int _{0}^{4}{\sqrt {\frac {4x+1}{4}}}dx=2\pi \int _{0}^{4}{\frac {1}{2}}\cdot {\sqrt {4x+1}}dx=\pi \int _{0}^{4}{\sqrt {4x+1}}dx=\pi \int _{0}^{4}{\sqrt {4x+1}}{\frac {d(2x+p)}{2}}={\frac {\pi }{2}}\cdot {\frac {\sqrt {(4x+1)^{3}}}{\frac {3}{2}}}|_{0}^{4}=}
=
π
2
⋅
2
3
⋅
(
(
4
⋅
4
+
1
)
3
−
(
4
⋅
0
+
1
)
3
)
=
π
3
⋅
(
17
3
−
1
)
=
π
3
⋅
(
4913
−
1
)
=
π
3
⋅
69.09279564
=
72.35380639
,
{\displaystyle ={\frac {\pi }{2}}\cdot {\frac {2}{3}}\cdot ({\sqrt {(4\cdot 4+1)^{3}}}-{\sqrt {(4\cdot 0+1)^{3}}})={\frac {\pi }{3}}\cdot ({\sqrt {17^{3}}}-1)={\frac {\pi }{3}}\cdot ({\sqrt {4913}}-1)={\frac {\pi }{3}}\cdot 69.09279564=72.35380639,}
kur d(4x+1)=4*dx; dx=(d(4x+1))/2.
Apskaičiuoti paraboloido paviršiaus plotą, atsiradusio dėl sukimo aplink Ox ašį parabolės
y
2
=
x
{\displaystyle y^{2}=x}
x kitimosi nuo
x
=
0
{\displaystyle x=0}
x
=
16
{\displaystyle x=16}
y
=
x
,
y
′
=
1
2
x
.
{\displaystyle y={\sqrt {x}},\quad y'={\frac {1}{2{\sqrt {x}}}}.}
S
=
2
π
∫
a
b
f
(
x
)
1
+
(
f
′
(
x
)
)
2
d
x
=
2
π
∫
0
16
x
1
+
(
1
2
x
)
2
d
x
=
2
π
∫
0
16
x
1
+
1
4
x
d
x
=
2
π
∫
0
16
x
4
x
+
1
4
x
d
x
=
{\displaystyle S=2\pi \int _{a}^{b}f(x){\sqrt {1+(f'(x))^{2}}}dx=2\pi \int _{0}^{16}{\sqrt {x}}{\sqrt {1+({\frac {1}{2{\sqrt {x}}}})^{2}}}dx=2\pi \int _{0}^{16}{\sqrt {x}}{\sqrt {1+{\frac {1}{4x}}}}dx=2\pi \int _{0}^{16}{\sqrt {x}}{\sqrt {\frac {4x+1}{4x}}}dx=}
=
2
π
∫
0
16
4
x
+
1
4
d
x
=
2
π
∫
0
16
1
2
⋅
4
x
+
1
d
x
=
π
∫
0
16
4
x
+
1
d
x
=
π
∫
0
16
4
x
+
1
d
(
4
x
+
1
)
4
=
π
4
⋅
(
4
x
+
1
)
3
3
2
|
0
16
=
{\displaystyle =2\pi \int _{0}^{16}{\sqrt {\frac {4x+1}{4}}}dx=2\pi \int _{0}^{16}{\frac {1}{2}}\cdot {\sqrt {4x+1}}dx=\pi \int _{0}^{16}{\sqrt {4x+1}}dx=\pi \int _{0}^{16}{\sqrt {4x+1}}{\frac {d(4x+1)}{4}}={\frac {\pi }{4}}\cdot {\frac {\sqrt {(4x+1)^{3}}}{\frac {3}{2}}}|_{0}^{16}=}
=
π
4
⋅
2
3
⋅
(
(
4
⋅
16
+
1
)
3
−
(
4
⋅
0
+
1
)
3
)
=
π
6
⋅
(
(
64
+
1
)
3
−
1
)
=
π
6
⋅
(
65
3
−
1
)
=
π
6
⋅
(
274625
−
1
)
=
π
6
⋅
523.0467536
=
273.8666398
,
{\displaystyle ={\frac {\pi }{4}}\cdot {\frac {2}{3}}\cdot ({\sqrt {(4\cdot 16+1)^{3}}}-{\sqrt {(4\cdot 0+1)^{3}}})={\frac {\pi }{6}}\cdot ({\sqrt {(64+1)^{3}}}-1)={\frac {\pi }{6}}\cdot ({\sqrt {65^{3}}}-1)={\frac {\pi }{6}}\cdot ({\sqrt {274625}}-1)={\frac {\pi }{6}}\cdot 523.0467536=273.8666398,}
kur d(4x+1)=4*dx; dx=(d(4x+1))/4.
S
=
2
π
∫
a
b
f
(
x
)
1
+
(
f
′
(
x
)
)
2
d
x
=
2
π
∫
0
4
x
2
1
+
(
2
x
)
2
d
x
=
2
π
∫
0
4
x
2
1
+
4
x
2
d
x
=
{\displaystyle S=2\pi \int _{a}^{b}f(x){\sqrt {1+(f'(x))^{2}}}dx=2\pi \int _{0}^{4}x^{2}{\sqrt {1+(2x)^{2}}}dx=2\pi \int _{0}^{4}x^{2}{\sqrt {1+4x^{2}}}dx=}
=
2
π
64
(
2
4
x
2
+
1
(
8
x
3
+
x
)
−
sinh
−
1
(
2
x
)
)
|
0
4
=
{\displaystyle ={\frac {2\pi }{64}}\left(2{\sqrt {4x^{2}+1}}(8x^{3}+x)-\sinh ^{-1}(2x)\right)|_{0}^{4}=}
=
π
32
(
2
65
⋅
516
−
sinh
−
1
8
)
−
π
32
(
2
1
⋅
0
−
sinh
−
1
0
)
=
{\displaystyle ={\frac {\pi }{32}}\left(2{\sqrt {65}}\cdot 516-\sinh ^{-1}8\right)-{\frac {\pi }{32}}\left(2{\sqrt {1}}\cdot 0-\sinh ^{-1}0\right)=}
=
π
32
(
8320.24999624410324
−
2.77647228072371767
)
−
π
32
(
0
−
0
)
=
{\displaystyle ={\frac {\pi }{32}}\left(8320.24999624410324-2.77647228072371767\right)-{\frac {\pi }{32}}\left(0-0\right)=}
=
π
32
⋅
8317.47352396337952
−
0
=
816.5660537284675687
=
{\displaystyle ={\frac {\pi }{32}}\cdot 8317.47352396337952-0=816.5660537284675687=}
=816.56605372846756868187758913704 (visiškai tiksliai).
Šitą plotą apskaičiavus su "Free Pascal 3.2.0" naudojant tokį kodą: var a:longint; c:real;
begin
for a:=1 to 1000000000 do
c:=c+sqrt(sqr(0.000000004)+sqr(sqr(0.000000004*a)-sqr(0.000000004*(a-1))))*sqr(0.000000004*a);
writeln(6.283185307179586477*c);
readln;
end.
gauname rezultatą "8.16566054823987990563E+0002", kuris reiškia 816.566054823987990563 (šiaip "FP 3.2.0" duoda rezultatą 64 bitų t. y. 17 skaitmenų tikslumu, bet dėl to, kad padauginta tik vieną kartą iš 19 skaitmenų turinčios
2
π
{\displaystyle 2\pi }
Apskaičiuosime plotą S , paviršiaus, gauto sukimu vienos arkos cikloidės
x
=
a
(
t
−
sin
t
)
,
{\displaystyle x=a(t-\sin t),}
y
=
a
(
1
−
cos
t
)
,
{\displaystyle y=a(1-\cos t),}
0
≤
t
≤
2
π
,
{\displaystyle 0\leq t\leq 2\pi ,}
Ox . Pagal antrą formulę turime
S
=
2
π
∫
0
2
π
a
(
1
−
cos
t
)
(
a
(
1
−
cos
t
)
)
2
+
(
a
sin
t
)
2
d
t
=
{\displaystyle S=2\pi \int _{0}^{2\pi }a(1-\cos t){\sqrt {(a(1-\cos t))^{2}+(a\sin t)^{2}}}dt=}
=
2
π
∫
0
2
π
a
(
1
−
cos
t
)
a
2
(
1
−
2
cos
t
+
cos
2
t
)
+
a
2
sin
2
t
d
t
=
2
π
∫
0
2
π
a
(
1
−
cos
t
)
a
2
(
2
−
2
cos
t
)
d
t
=
{\displaystyle =2\pi \int _{0}^{2\pi }a(1-\cos t){\sqrt {a^{2}(1-2\cos t+\cos ^{2}t)+a^{2}\sin ^{2}t}}dt=2\pi \int _{0}^{2\pi }a(1-\cos t){\sqrt {a^{2}(2-2\cos t)}}dt=}
=
2
2
π
a
2
∫
0
2
π
(
1
−
cos
t
)
3
2
d
t
=
2
2
π
a
2
∫
0
2
π
(
2
sin
2
t
2
)
3
2
d
t
=
8
π
a
2
∫
0
2
π
sin
3
t
2
d
t
=
{\displaystyle =2{\sqrt {2}}\pi a^{2}\int _{0}^{2\pi }(1-\cos t)^{3 \over 2}dt=2{\sqrt {2}}\pi a^{2}\int _{0}^{2\pi }(2\sin ^{2}{t \over 2})^{3 \over 2}dt=8\pi a^{2}\int _{0}^{2\pi }\sin ^{3}{t \over 2}dt=}
=
32
π
a
2
∫
0
π
2
sin
3
t
2
d
(
t
2
)
=
32
π
a
2
⋅
2
!
!
3
!
!
=
64
3
π
a
2
,
{\displaystyle =32\pi a^{2}\int _{0}^{\pi \over 2}\sin ^{3}{t \over 2}d({t \over 2})=32\pi a^{2}\cdot {2!! \over 3!!}={64 \over 3}\pi a^{2},}
kur pasinaudojome dvigubu faktorialu .
Arba galima buvo šį integralą sudorot paprastai:
S
=
8
π
a
2
∫
0
2
π
sin
3
t
2
d
t
=
8
π
a
2
∫
0
2
π
3
sin
t
2
−
sin
3
t
2
4
d
t
=
2
π
a
2
∫
0
2
π
(
3
sin
t
2
−
sin
3
t
2
)
d
t
=
{\displaystyle S=8\pi a^{2}\int _{0}^{2\pi }\sin ^{3}{t \over 2}dt=8\pi a^{2}\int _{0}^{2\pi }{3\sin {t \over 2}-\sin {3t \over 2} \over 4}dt=2\pi a^{2}\int _{0}^{2\pi }(3\sin {t \over 2}-\sin {3t \over 2})dt=}
=
4
π
a
2
∫
0
2
π
3
sin
t
2
d
(
t
2
)
−
4
3
π
a
2
∫
0
2
π
sin
3
t
2
d
(
3
t
2
)
=
−
12
π
a
2
cos
t
2
|
0
2
π
+
4
3
π
a
2
cos
3
t
2
|
0
2
π
=
{\displaystyle =4\pi a^{2}\int _{0}^{2\pi }3\sin {t \over 2}d({t \over 2})-{4 \over 3}\pi a^{2}\int _{0}^{2\pi }\sin {3t \over 2}d({3t \over 2})=-12\pi a^{2}\cos {t \over 2}|_{0}^{2\pi }+{4 \over 3}\pi a^{2}\cos {3t \over 2}|_{0}^{2\pi }=}
=
−
12
π
a
2
⋅
(
−
2
)
+
4
3
π
a
2
⋅
(
−
2
)
=
64
3
π
a
2
.
{\displaystyle =-12\pi a^{2}\cdot (-2)+{4 \over 3}\pi a^{2}\cdot (-2)={64 \over 3}\pi a^{2}.}
V
=
π
∫
a
b
y
2
d
x
=
π
∫
a
b
[
f
(
x
)
]
2
d
x
.
{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{a}^{b}[f(x)]^{2}\;dx.}
Funkcija sukama aplink Ox ašį. Apskaičiuosime paraboloido
y
=
x
{\displaystyle y={\sqrt {x}}}
V
=
π
∫
a
b
y
2
d
x
=
π
∫
0
16
(
x
)
2
d
x
=
π
∫
0
16
x
d
x
=
π
∫
0
16
x
d
x
=
π
⋅
x
2
2
|
0
16
=
π
(
16
2
2
−
0
2
2
)
=
π
⋅
256
2
=
128
π
=
402.1238597.
{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{0}^{16}({\sqrt {x}})^{2}dx=\pi \int _{0}^{16}x\;dx=\pi \int _{0}^{16}x\;dx=\pi \cdot {\frac {x^{2}}{2}}|_{0}^{16}=\pi ({\frac {16^{2}}{2}}-{\frac {0^{2}}{2}})=\pi \cdot {\frac {256}{2}}=128\pi =402.1238597.}
Apskaičiuosime kubinio paraboloido
y
=
x
3
{\displaystyle y={\sqrt[{3}]{x}}}
y
=
x
3
{\displaystyle y={\sqrt[{3}]{x}}}
Ox ašį ir tokiu budu gaunamas kubinio paraboloido tūris.
V
=
π
∫
a
b
y
2
d
x
=
π
∫
0
16
(
x
3
)
2
d
x
=
π
∫
0
16
x
2
3
d
x
=
π
⋅
x
2
+
3
3
5
3
|
0
16
=
π
⋅
3
5
⋅
x
5
3
|
0
16
=
π
⋅
3
5
⋅
(
16
5
3
−
0
5
3
)
=
{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{0}^{16}({\sqrt[{3}]{x}})^{2}dx=\pi \int _{0}^{16}x^{\frac {2}{3}}\;dx=\pi \cdot {\frac {x^{2+3 \over 3}}{5 \over 3}}|_{0}^{16}=\pi \cdot {\frac {3}{5}}\cdot x^{5 \over 3}|_{0}^{16}=\pi \cdot {\frac {3}{5}}\cdot ({\sqrt[{3}]{16^{5}}}-{\sqrt[{3}]{0^{5}}})=}
=
π
⋅
3
5
⋅
1048576
3
=
π
⋅
3
5
⋅
101.5936673
=
60.9562004
π
=
191.4995514.
{\displaystyle =\pi \cdot {\frac {3}{5}}\cdot {\sqrt[{3}]{1048576}}=\pi \cdot {\frac {3}{5}}\cdot 101.5936673=60.9562004\pi =191.4995514.}
Apskaičiuosime funkcijos
y
=
x
2
{\displaystyle y=x^{2}}
x kinta nuo 0 iki 4.
V
=
π
∫
a
b
y
2
d
x
=
π
∫
0
4
(
x
2
)
2
d
x
=
π
∫
0
4
x
4
d
x
=
π
⋅
x
5
5
|
0
4
=
π
(
4
5
5
−
0
5
5
)
=
π
⋅
1024
5
=
204.8
π
=
643.3981755.
{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{0}^{4}(x^{2})^{2}dx=\pi \int _{0}^{4}x^{4}\;dx=\pi \cdot {\frac {x^{5}}{5}}|_{0}^{4}=\pi ({\frac {4^{5}}{5}}-{\frac {0^{5}}{5}})=\pi \cdot {\frac {1024}{5}}=204.8\pi =643.3981755.}
Apskaičiuosime funkcijos
y
=
x
3
{\displaystyle y=x^{3}}
x kinta nuo 0 iki 4.
V
=
π
∫
a
b
y
2
d
x
=
π
∫
0
4
(
x
3
)
2
d
x
=
π
∫
0
4
x
6
d
x
=
π
⋅
x
7
7
|
0
4
=
π
(
4
7
7
−
0
7
7
)
=
π
⋅
16384
7
=
2340.571429
π
=
7353.122005.
{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{0}^{4}(x^{3})^{2}dx=\pi \int _{0}^{4}x^{6}\;dx=\pi \cdot {\frac {x^{7}}{7}}|_{0}^{4}=\pi ({\frac {4^{7}}{7}}-{\frac {0^{7}}{7}})=\pi \cdot {\frac {16384}{7}}=2340.571429\pi =7353.122005.}

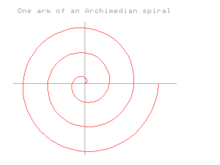






![{\displaystyle t\in [t_{0};T],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0b874c1b132c7f3e6239aa445b37e2d8d961fd2c)



![{\displaystyle t\in [t_{0};T]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c296cc887890e455320a28f1796e1e443e9be296)


![{\displaystyle \phi \in [\alpha ;\beta ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2007849b5cdd53dbc01143bff5739409c7e1efd9)















![{\displaystyle (x\in [3;8])}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9e617ed2a41956e13516c5e05a33a02bd1eb6ab6)














![{\displaystyle =4[{243 \over 5}-9-({32 \over 5}-{8 \over 3})]=4({211 \over 5}-{19 \over 3})={2152 \over 15}\;(g)=143.4(6)\;(g),}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f7d0edc2da49e522bb6c0c01ac24357d8fe961b1)























































![{\displaystyle \phi \in [0;\pi ].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f19a9597e1cc810ca74f674ba9e726c58f25cb34)




















![{\displaystyle s=\int _{\alpha }^{\beta }{\sqrt {1+\left[{\frac {\psi '(t)}{\phi '(t)}}\right]^{2}}}\phi '(t)dt=\int _{\alpha }^{\beta }{\sqrt {[\phi '(t)]^{2}+\left[\psi '(t)\right]^{2}}}dt.\quad (5)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/52d3f404adfe890a77889b3e006aed5a63451f8f)







![{\displaystyle \left({\frac {dx}{d\theta }}\right)^{2}+\left({\frac {dy}{d\theta }}\right)^{2}=[f'(\theta )]^{2}+[f(\theta )]^{2}=\rho '^{2}+\rho ^{2}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/79191333e257361190607ea880e97541820e1e70)

![{\displaystyle \left({\frac {dx}{d\theta }}\right)^{2}=(f'(\theta )\cos \theta -f(\theta )\sin \theta )^{2}=[f'(\theta )]^{2}\cos ^{2}\theta -2f'(\theta )\cos \theta f(\theta )\sin \theta +[f(\theta )]^{2}\sin ^{2}\theta ;}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ace2f1d8fb73ba0786f20daacce8c148fe2c9b5f)
![{\displaystyle \left({\frac {dy}{d\theta }}\right)^{2}=(f'(\theta )\sin \theta +f(\theta )\cos \theta )^{2}=[f'(\theta )]^{2}\sin ^{2}\theta +2f'(\theta )\sin \theta f(\theta )\cos \theta +[f(\theta )]^{2}\cos ^{2}\theta .}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a08efd17279343c413a255f9d5c8e8d44351fbdb)
























![{\displaystyle ={{4 \over 9}(1+{9x \over 4})^{3 \over 2} \over {3 \over 2}}|_{0}^{5}={8 \over 27}(1+{9x \over 4})^{3 \over 2}|_{0}^{5}={8 \over 27}[({4 \over 4}+{45 \over 4})^{3 \over 2}-(1+0)^{3 \over 2}]={8 \over 27}[({7 \over 2})^{3}-1]={335 \over 27}\approx 12,4074;}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cc9522e8da69a1155319393fac197a3cae8d87b9)








![{\displaystyle ={4 \over 9}\cdot {(1+{9x \over 4})^{3 \over 2} \over {3 \over 2}}|_{1}^{5}={8 \over 27}(1+{9x \over 4})^{3 \over 2}|_{1}^{5}={8 \over 27}[({4 \over 4}+{45 \over 4})^{3 \over 2}-(1+{9 \over 4})^{3 \over 2}]={8 \over 27}[({7 \over 2})^{3}-{\sqrt {(1+2,25)^{3}}}]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f61978280c81f73d8c3bc87814e93e6cba28d06a)









































































































![{\displaystyle ={2 \over 18\cdot 3}{\sqrt {(13+9x)^{3}}}|_{4}^{12}={1 \over 27}[{\sqrt {(13+9\cdot 12)^{3}}}-{\sqrt {(13+9\cdot 4)^{3}}}]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31f0a3eaa5b723d46ad5df8cdbd6098561b5407b)
![{\displaystyle ={1 \over 27}[{\sqrt {1771561}}-{\sqrt {117649}}]={1 \over 27}\cdot (1331-343)={988 \over 27}=\approx 36,59259259.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/68dfa79a99dc50eee85a9497807734ba79e800fd)


![{\displaystyle c={\sqrt {(12-4)^{2}+[{\sqrt {(12+1)^{3}}}-{\sqrt {(4+1)^{3}}}]^{2}}}=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/05e1d8c98fb605421c07fad6bc2d0387016ee95a)





![{\displaystyle ={1 \over 2}[x{\sqrt {{1 \over x}+4}}+{1 \over 4}\ln(4x({\sqrt {{1 \over x}+4}}+2)+1)]|_{1}^{16}=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7aa4669266b216a16fdc24fb7efbe6fbca2f480d)
![{\displaystyle ={1 \over 2}[16{\sqrt {{1 \over 16}+4}}+{1 \over 4}\ln(4\cdot 16({\sqrt {{1 \over 16}+4}}+2)+1)]-{1 \over 2}[1{\sqrt {{1 \over 1}+4}}+{1 \over 4}\ln(4({\sqrt {{1 \over 1}+4}}+2)+1)]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c9a2f879f22d8ec09324641e912182a102878bb1)
![{\displaystyle ={1 \over 2}[16\cdot 2,015564437+{1 \over 4}\ln(64(2,015564437+2)+1)]-{1 \over 2}[{\sqrt {5}}+{1 \over 4}\ln(4({\sqrt {5}}+2)+1)]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/720b1aeffabe7b165858577415ce6f0b44fe89d3)
![{\displaystyle ={1 \over 2}[32,24903099+{1 \over 4}\ln(256,996124+1)]-{1 \over 2}[2,236067978+{1 \over 4}\ln(17,94427191)]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d15f6badc75e2aebcaefa2d70945fc7da99af11c)
![{\displaystyle ={1 \over 2}[32,24903099+{1 \over 4}\cdot 5,552944561]-{1 \over 2}[2,236067978+{2,88727095 \over 4}]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4a643a9b0680fecc99fe5aa015e03599b381a2d0)











![{\displaystyle t\in [0;2\pi ].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7985df1d8dbe135ba76cdaecafb3bc618370241b)





















![{\displaystyle =a[\pi {\sqrt {4\pi ^{2}+1}}+{1 \over 2}\ln(2\pi +{\sqrt {4\pi ^{2}+1}})]=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/16ebe9f43af89e77b7e02823a6e97c252b9798b9)
![{\displaystyle =a[19,9876454+1,268648751]=a21,25629415.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/571ff0e4cf4224fa81fa6f947335ca5bef28d49c)















![{\displaystyle m=\int _{L}\gamma {\sqrt {1+[y']^{2}}}\;dx=\int _{0}^{5}{\sqrt {x^{2}+y^{2}}}{\sqrt {1+[y']^{2}}}\;dx=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/22e88773ce0d7fc6b0c1dca842c7a19b69d5dd6c)




















![{\displaystyle m=\int _{0}^{5}{\sqrt {x^{2}+y^{2}}}{\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}{\sqrt {x^{2}+(x^{2})^{2}}}{\sqrt {1+(2x)^{2}}}dx,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bbe481bd7f13080ddd7035c7ff58392c14db3cb7)






![{\displaystyle m=\int _{0}^{10}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{10}x{\sqrt {1+(2x)^{2}}}dx=\int _{0}^{10}x{\sqrt {1+4x^{2}}}dx=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b56e13098551a0566a29f08dc923fad4df5e7f06)




![{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}x{\sqrt {1+4x^{2}}}dx=2\int _{0}^{5}x{\sqrt {{\frac {1}{4}}+x^{2}}}dx=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c235d2f0757c3838de8c29cbceed5f82b81fffd7)


![{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}x{\sqrt {1+4x^{2}}}dx}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e214cdf54c280b4f38cf26b8b9540ded87e12737)



![{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+y){\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2}){\sqrt {1+4x^{2}}}dx=2\int _{0}^{5}x(1+x){\sqrt {{\frac {1}{4}}+x^{2}}}dx;}](https://wikimedia.org/api/rest_v1/media/math/render/svg/951f72fc747d26b10e29021aea84c6f256425278)






![{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+y)^{2}{\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2})^{2}{\sqrt {1+4x^{2}}}dx=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/770d7a9525f494720dcd8e265c40c4d9a16ae00d)





![{\displaystyle m=\int _{0}^{5}\gamma {\sqrt {1+[y']^{2}}}dx=\int _{0}^{5}(x+x^{2})^{2}{\sqrt {1+4x^{2}}}dx}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2225a735a9015ec999e74b29e6c41e7931a8d098)










![{\displaystyle [\alpha ,\beta ],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5ecd887db27c26326f61524a15317372d0ef60fa)






























![{\displaystyle =2\pi {\sqrt {p}}\int _{0}^{k}{\sqrt {2x+p}}dx=\pi {\sqrt {p}}\int _{0}^{k}{\sqrt {2x+p}}d(2x+p)=\pi {\sqrt {p}}\cdot {\frac {\sqrt {(2x+p)^{3}}}{3/2}}|_{0}^{k}={\frac {2\pi {\sqrt {p}}}{3}}\cdot [(2k+p)^{3 \over 2}-p^{3 \over 2}],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d26a0c3062b5ecb0a14de9d20c2a8a998d7f203e)
























![{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{a}^{b}[f(x)]^{2}\;dx.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/775d658e2c588b7e09031505c3b12bc868db8ef4)

![{\displaystyle y={\sqrt[{3}]{x}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/be50c0a49b200fb46800951d0268b0a9d4e3fdda)
![{\displaystyle V=\pi \int _{a}^{b}y^{2}\;dx=\pi \int _{0}^{16}({\sqrt[{3}]{x}})^{2}dx=\pi \int _{0}^{16}x^{\frac {2}{3}}\;dx=\pi \cdot {\frac {x^{2+3 \over 3}}{5 \over 3}}|_{0}^{16}=\pi \cdot {\frac {3}{5}}\cdot x^{5 \over 3}|_{0}^{16}=\pi \cdot {\frac {3}{5}}\cdot ({\sqrt[{3}]{16^{5}}}-{\sqrt[{3}]{0^{5}}})=}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ff8823fd9ea5b8ebc4a3fd06e288d0d05f05291b)
![{\displaystyle =\pi \cdot {\frac {3}{5}}\cdot {\sqrt[{3}]{1048576}}=\pi \cdot {\frac {3}{5}}\cdot 101.5936673=60.9562004\pi =191.4995514.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9a55da54d6e260f634aac61d98d210b3f21b2d4b)


